It All Adds Up

However, before it became a subject for great minds, geometry gained its place on the ground. Its etymology bears witness to this: it is first and foremost the science of the measurement of the Earth, and the first surveyors were hands-on mathematicians. Problems concerning the division of territory were then classics of the craft. How to divide a field into equal parts? How to determine the price of a plot of land from its area? Which of two plots is closer to the river? What route should the future canal follow to make it the shortest possible?

All these questions were paramount in ancient societies where the whole economy still revolved in a vital way around agriculture and hence around the distribution of land. In response to this, geometrical know-how was built up, enriched and transmitted from generation to generation. Anyone equipped with this know-how was certain to hold a central and indisputable place in society.

For these measurement professionals, the rope was often the primary instrument of geometry. In Egypt, ‘ropestretcher’ was a profession in its own right. When the Nile floods led to regular inundations, it was the ropestretchers who were sent for to redefine the boundaries of plots that bordered the river. Using information they recorded about the ground, they planted their stakes, stretched their long ropes across the fields, and then carried out calculations that enabled them to rediscover the boundaries erased by the floodwaters.

They were also the first port of call in constructing buildings, when they took the measurements on the ground and marked the precise location of the building based on architects’ plans. And in the case of a temple or an important monument, it was often the pharaoh in person who symbolically came to stretch the first rope.

It can be said that the rope was the all-purpose tool of geometry. Surveyors used it as a ruler, as compasses, and as a set-square.

To use it as a ruler is straightforward: if you stretch the rope between two fixed points you obtain a straight line. And if you require a graduated ruler, you just tie knots at regular intervals along your rope. For compasses, there is no magic involved either. You simply fix one of the two ends to a stake, stretch the rope and move the other end around the stake. This gives a circle. And if your rope is graduated, you can control the length of the radius exactly.

For the set-square, however, things are slightly more complicated. Let’s look at this particular problem for a few moments: what would you do to draw a right angle? With a bit of research, one can come up with several different methods. If, for example, you draw two circles that intersect each other, then the straight line that joins their centres is perpendicular to the straight line that passes through their two points of intersection. There is your right angle.

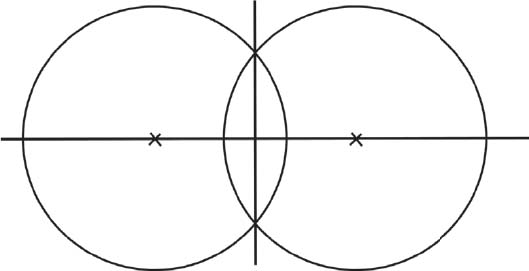
From a theoretical point of view, this construction works perfectly, but things are more complicated in practice. Imagine the surveyors, out in the fields, having to lay out two large circles precisely every time they needed to draw a right angle or, more simply, to verify that an angle which had already been constructed was actually a right angle. This was neither fast nor efficient.

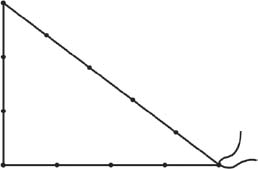
The surveyors adopted a different method, which was subtler and more practical: they used their rope directly to form a triangle with a right angle (known as a right-angled triangle). The most famous one is the 3–4–5. If you take a rope divided into twelve intervals by thirteen knots, then you can form a triangle whose sides measure three, four and five intervals, respectively. And, as if by magic, the angle formed by the sides of length 3 and 4 is a perfect right angle.

Four thousand years ago, the Babylonians already had tables of numbers that could be used to construct right-angled triangles. The Plimpton 322 Tablet, which is currently in the collections of Columbia University in New York City, and dates from 1800 BC, contains a table of fifteen triples of such numbers (so-called Pythagorean triples). Apart from the 3-4-5, it has fourteen other triangles, some of which are considerably more complicated, such as the 65-72-97 or even the 1,679-2,400-2,929. Up to a few minor mistakes, such as errors in calculation or transcription, the triangles of the Plimpton Tablet are perfectly exact, and they all have a right angle.

It is difficult to know the precise period from which the Babylonian surveyors began to use their knowledge of right-angled triangles on the ground, but the use of these triangles persisted well beyond the disappearance of the Babylonian civilization. In the Middle Ages, for example, the rope with thirteen knots remained an essential tool for cathedral builders.

On our journey through the history of mathematics, it is by no means uncommon to find certain similar ideas appearing independently at removes of thousands of kilometres and in profoundly different cultural contexts. One such startling coincidence is that during the first millennium BC the Chinese civilization developed a whole mathematical know-how that corresponds remarkably to that of the Babylonian, Egyptian and Greek civilizations of the same period.

This knowledge was amassed over the centuries before being compiled under the Han dynasty, around 2,200 years ago, into one of the world’s first great mathematical works: The Nine Chapters on the Mathematical Art.

The first of these Nine Chapters is entirely devoted to the study of measurements of fields of various shapes. Rectangles, triangles, trapezia, circles, portions of circles and also rings represent geometric figures for which procedures for calculating their areas are described in minute detail. Later in the work, you discover that the ninth and final chapter deals with right-angled triangles. And guess which figure is discussed from the very first sentence of this chapter: the 3-4-5!

Good ideas are like that. They transcend cultural differences and are able to blossom spontaneously wherever human minds are ready to devise and absorb them.

SOME PROBLEMS OF THE PERIOD

Questions about fields, about architecture, or more generally about land planning led the scholars of antiquity to set themselves a great diversity of geometric problems of which the following are examples.

The following statement, taken from the Babylonian Tablet BM 85200, shows that the Babylonians were not content with plane geometry, but also thought in the space dimension.

An excavation. So much as the length, that is the depth. 1 the earth I have torn out. My ground and the earth I have accumulated, 1´10. Length and width: '50. Length and width, what?1

As you will have gathered, the style of the mathematicians of Babylon was telegraphic in nature. If we expand it further, this same statement might look as follows:

The depth of an excavation is twelve times greater than its length.2 If i dig further so that my excavation has one more unit of depth, then its volume will be equal to 7/6. If i add the length to the width i obtain 5/6.3 What are the dimensions of the excavation?

This problem was accompanied by the detailed method for solving it, ending with the solution: the length is ½, the width ⅓ and the depth 6.

Let’s now take a short trip down the Nile. As a matter of course, in the case of the Egyptians, we find problems about pyramids. The following statement is an extract from a famous papyrus, the Rhind papyrus, copied by the scribe Ahmes, dating from the first half of the sixteenth century BC.

A pyramid has a base side of 140 cubits and an inclination 4 of 5 palms and 1 digit, what is its altitude?’

The cubit, the palm and the digit were units of measurement of 52.5 centimetres, 7.5 centimetres and 1.88 centimetres, respectively. Ahmes also gave the solution: 93 + ⅓ cubits. In this same papyrus, the scribe also took on the geometry of the circle.

Example of the calculation of a round field with a diameter of 9 khet. What is the value of its area?

The khet is also a unit of measurement representing approximately 52.5 metres. To solve this problem, Ahmes stated that the area of this circular field is equal to that of a square field with a side of length 8 khet. The comparison is extremely useful, for it is much easier to calculate the area of a square than that of a circle. His solution was 8 × 8 = 64. However, the mathematicians who succeeded Ahmes came to discover that his result was not quite exact. The areas of the circle and the square do not quite agree. Since then, many people have attempted to answer the question: how do you construct a square with an area equal to that of a given circle? Many have worn themselves out in vain in this pursuit, but for a reason. Without knowing it, Ahmes was one of the first to tackle what would become one of history’s classic mathematical conundrums: the squaring of the circle.

In China too, people sought to calculate the area of circular fields. The following problem is taken from the first of the Nine Chapters.

Suppose one has a circular field with circumference 30 bu and diameter 10 bu. The question is how big is the field?5

Here, a bu is equal to about 1.4 metres. And, as in Egypt, the mathematicians kept tripping over the rug with this figure. The original statement was already known to be false, since a circle of diameter 10 has a circumference slightly greater than 30. However, that did not prevent Chinese scholars from putting an approximate value on the area (75 bu), or from complicating the task further for themselves by continuing on to questions about circular rings!

Suppose one has a field in the shape of a ring with internal circumference 92 bu, external circumference 122 bu, and transverse diameter 5 bu. The question is: how big is the field?

It seems likely that there were never any ring-shaped fields in ancient China – these latter problems suggest that the scholars of the Central Kingdom were into geometry and raised these questions as purely theoretical challenges. Research into ever more improbable and weird-looking geometric figures in order to study and understand them remains a favourite pastime of our mathematicians to this day.

Among the ranks of professional geometers, one must also include the Bematists. While it was the job of surveyors and other ropestretchers to measure fields and buildings, the Bematists had a much grander view of things. In Greece, it was the job of these men to measure long distances by counting their steps.

And sometimes their work could take them a long way from home. For example, in the fourth century BC, Alexander the Great took several Bematists with him on his campaign in Asia, which led him as far as the boundaries of what is now India. These walking geometers thus had to measure routes of several thousand kilometres in length.

Step back a little and imagine for a moment the strange spectacle of these men walking in quick time, traversing the immense landscapes of the Middle East. See them cross the plateaux of Upper Mesopotamia; walk through the arid yellow settings of the Sinai Peninsula to reach the fertile banks of the Nile Valley; then turn back, setting off to brave the mountainous massifs of the Persian Empire and the deserts of what is now Afghanistan. Can you see them, calmly walking on and on, with a dry and monotonous rhythm, and passing through the foothills of the gigantic mountains of the Hindu Kush to return via the shores of the Indian Ocean? And all the while they counted their paces tirelessly.

The image is captivating, and the outrageous challenge of their undertaking seems insane. And yet, their results were remarkably accurate: less than 5 per cent difference on average between their measurements and the true distances we know today. Alexander’s Bematists thus made it possible to describe the geography of his kingdom in a way that had never before been achieved for so vast a region.

Two centuries later, in Egypt, a scholar of Greek origin named Eratosthenes conceived an even larger project – that of measuring nothing less than the circumference of the Earth. Of course, there was no question of sending poor Bematists on a tour of the planet. However, based on skilful observations of the difference in the inclination of the sun’s rays between the towns of Syene (the present-day Aswan) and Alexandria, Eratosthenes calculated that the distance between the two towns must represent one fiftieth of the total circumference of the Earth.

Naturally, he then called upon the Bematists to undertake the measurement. Unlike their Greek counterparts, the Egyptian Bematists did not count their own steps, but those of a camel they took with them. The animal is renowned for the regularity of its walking. After long days of travelling along the Nile, the verdict was issued: the two towns were 5,000 stadia apart and the girth of our planet was therefore 250,000 stadia, or 39,375 kilometres. Once again, the result was astonishingly accurate, as we know today that this circumference measures exactly 40,008 kilometres. The error was less than 2 per cent.

Perhaps more than any other ancient people, the Greeks gave geometry pride of place in the heart of their culture. It was recognized for its rigour and its ability to form the mind. For Plato, it was an obligatory rite of passage for any budding philosopher, and legend has it that the slogan ‘Let no one ignorant of geometry enter’ was engraved at the entrance to his academy.

Geometry was so much in vogue that it eventually spilled over into other disciplines. The arithmetical properties of numbers were thus interpreted in geometric language. See, for example this definition by Euclid extracted from the seventh book of his Elements of Mathematics dating from the third century BC:

And when two numbers multiplying one another make some other number then the number so created is called plane, and its sides are the numbers which multiply one another.

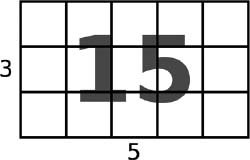
If I take the product 5 × 3, the numbers 5 and 3 are thus called, according to Euclid, the ‘sides’ of the multiplication. Why is this? It is simply because a multiplication can be represented as the area of a rectangle. If the latter has a width equal to 3 and a length of 5, its area is 5 × 3. The numbers 3 and 5 are precisely the sides of the rectangle. The result of the multiplication, 15, is for its part called the ‘plane’, since it corresponds geometrically to a surface.

Similar constructions relate to other geometric figures. For example, a number is said to be triangular if it can be represented in the form of a triangle. The first triangular numbers are 1, 3, 6 and 10.

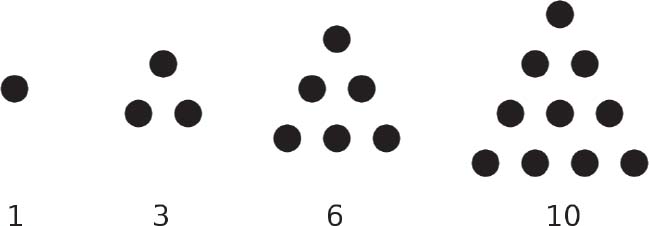
The last of the above triangles with ten points is nothing other than the famous tetractys that Pythagoras and his disciples employed as the symbol of the harmony of the cosmos. On the same principle, we also find the square numbers whose first representatives are 1, 4, 9 and 16.

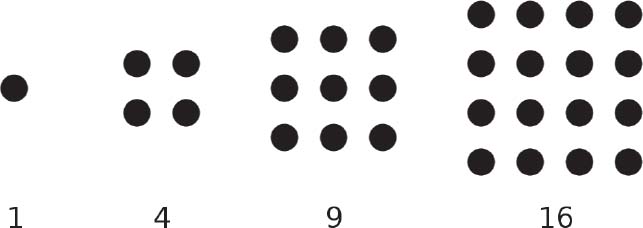
And we could of course continue for a long time like that with all kinds of figures. Thus, the geometric representation of numbers makes it possible to render visual and evident properties that are otherwise seemingly incomprehensible.

By way of example, have you ever tried adding the odd numbers successively, one after another: 1 + 3 + 5 + 7 + 9 + 11 + …? No? Well, something completely surprising happens. Look:

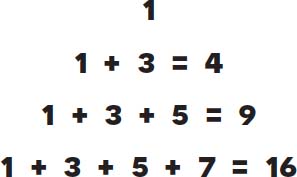
Can you see something special about the numbers that emerge? In the order in which they occur: 1, 4, 9, 16 … These are the square numbers!

And you can continue as long as you wish; this rule will never be broken. Add the first ten odd numbers from 1 to 19, if you are brave enough, and you will reach 100, which is the tenth square:

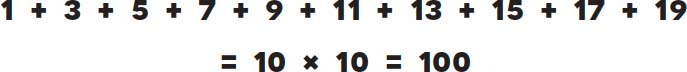
Isn’t that surprising? But why? What kind of miracle makes this property always hold? Of course, it would be possible to give a numerical proof, but there is a much simpler way. Using the geometrical representation, you just have to slice up the square numbers and the explanation becomes plain to see.

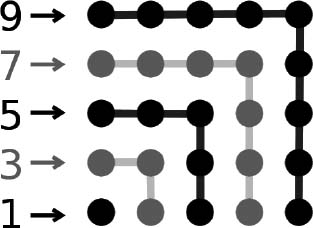
Each slice adds an odd number of balls, and increases the side of the square by one unit. This completes the simple and lucid proof.

In the kingdom of mathematics, geometry was the queen, and no assertion could be verified without coming under its scrutiny. Its reign lasted far beyond antiquity and the Greeks. It was almost two thousand years before the scholars of the Renaissance launched a vast movement to modernize mathematics which would dethrone geometry in favour of a completely new language: that of algebra.

4

THE AGE OF THEOREMS

It is the beginning of May. At midday the sun is shining over the Parc de la Villette in northern Paris. Opposite me towers the Cité des Sciences et de l’Industrie (City of Science and Industry), the largest science museum in Europe, with its Geode in the foreground. Built in the mid-1980s, this strange cinema of some 36 metres in diameter resembles a gigantic sphere with many facets.

This is very much a stopping-off place. There are tourists clutching their cameras – they have come to see this curious building in the French capital. There are families out for their Wednesday stroll. A few lovers are sitting on the grass or walking hand in hand. Here and there, a jogger zigzags through the tide of local residents who pass through with a show of indifference, scarcely casting a glance towards the strange apparition of this shimmering sphere in the middle of their day-to-day lives. All around the sphere, children amuse themselves by observing the distorted image of the surrounding world that it reflects.

As for me, if I am here today it is because I am especially interested in its geometry. I start to walk closer, scanning it closely. Its surface comprises thousands of triangular mirrors assembled together. At first sight the assembly may appear perfectly regular, but after a few minutes’ scanning I begin to notice several irregularities. At certain well-defined points, the triangles grow distorted and are stretched as if by a malformation of the structure. Although they are grouped together six per hexagon, forming a perfectly regular meshing almost everywhere on the sphere, there are a dozen special points where these triangles are only grouped in fives.

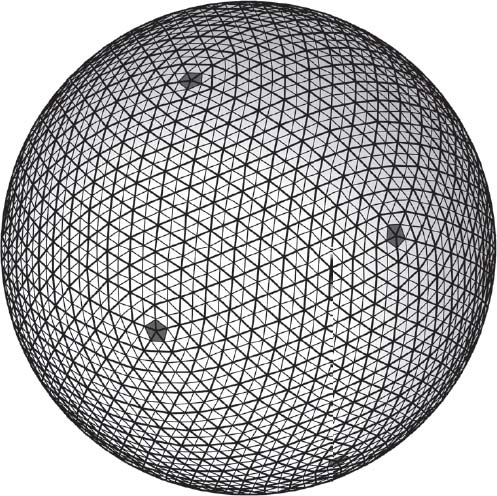
Representation of the Geode and its thousands of triangles. The points where the triangles occur in groups of five are shown in dark grey.

These irregularities are almost invisible at first glance. The fact is that most passers-by pay no attention to them. At the same time, as a mathematician, I do not see anything surprising in them. I even have to say that I expected to find them. The architect did not make a mistake; moreover, there are numerous other buildings elsewhere in the world with a similar geometry, and all have these same dozen points where the basic pieces are grouped together in fives rather than sixes. These points are the results of inescapable geometric constraints discovered over 2,000 years ago by the Greek mathematicians.

Theaetetus of Athens was a mathematician of the fourth century BC who is generally credited with the complete description of regular polyhedra. A polyhedron, in geometry, is simply a three-dimensional figure delimited by several plane faces. For example, cubes and pyramids belong to the family of polyhedra, unlike spheres and cylinders, which have rounded faces. The geode, with its triangular faces, can also be considered as a giant polyhedron, even though its large number of faces makes it resemble a sphere from a distance.

Theaetetus was especially interested in perfectly symmetric polyhedra, those for which all the faces and all the angles are the same. And his discovery was at least disconcerting: he only found five, and proved that there are no others. Five solids and that’s all – not a single one more.

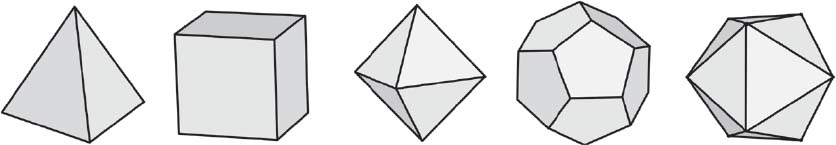
From left to right: the tetrahedron, the hexahedron, the octahedron, the dodecahedron and the icosahedron.

To this day, the polyhedra are usually named according to the number of their faces, written in Ancient Greek, followed by the suffix -hedron. For example, in geometry, the cube with its six square faces is referred to as the hexahedron. The tetrahedron, the octahedron, the dodecahedron and the icosahedron have four, eight, twelve and twenty faces, respectively. These five polyhedra subsequently became known as the Platonic solids.

Platonic? Why are they not associated in name with Theaetetus? History is sometimes unfair, and the discoverers are not always those who receive the honours of posterity. The Athenian philosopher Plato had nothing to do with the discovery of the five solids, but he made them famous through a theory that associated them with the elements of the cosmos: fire is associated with the tetrahedron, earth with the hexahedron, air with the octahedron and water with the icosahedron. As for the dodecahedron, with its pentagonal faces, Plato claimed that it was the shape of the Universe. This theory was abandoned by science a long time ago, and yet these five regular polyhedra are still conventionally associated with Plato.

As it happens, Theaetetus himself was not actually the first to discover these five solids. There exist considerably older sculpted models and written descriptions of them. For example, a collection of small sculpted stone balls reproducing the shapes of the Platonic solids came to light in Scotland and is said to date from one thousand years before Theaetetus. These pieces are currently conserved in the Ashmolean Museum in Oxford.

So is Theaetetus no more worthy than Plato? Is he also an impostor? He is not a total impostor, for while the five figures were known before his time, he was the first to demonstrate clearly that the list was complete. There is no point in looking any further, Theaetetus tells us, no one will ever find any more. This assertion has something reassuring about it. It removes a dreadful doubt. Phew – they’re all present and correct!

This step is emblematic of the way in which Greek mathematicians came to approach mathematics. For them, it was no longer a matter of simply finding solutions that worked. They wanted to exhaust the problem. They wanted to make sure that nothing escaped them. And for that reason, they developed the art of mathematical exploration to its peak.