
Полная версия
Fermat’s Last Theorem
From Pythagoras’ Theorem to Fermat’s Last Theorem
Pythagoras’ theorem and its infinity of triples was discussed in E.T. Bell’s The Last Problem, the library book which caught the attention of the young Andrew Wiles. Although the Brotherhood had achieved an almost complete understanding of Pythagorean triples, Wiles soon discovered that this apparently innocent equation, x2 + y2 = z2, has a darker side – Bell’s book described the existence of a mathematical monster.
In Pythagoras’ equation the three numbers, x, y and z, are all squared (i.e. x2 = x × x):
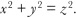
However, the book described a sister equation in which x, y and z are all cubed (i.e. x3 = x × x × x). The so-called power of x in this equation is no longer 2, but rather 3:
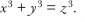
Finding whole number solutions, i.e. Pythagorean triples, to the original equation was relatively easy, but changing the power from ‘2’ to ‘3’ (the square to a cube) and finding whole number solutions to the sister equation appears to be impossible. Generations of mathematicians scribbling on notepads have failed to find numbers which fit the equation perfectly.
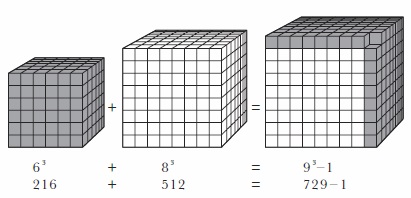
Figure 4. Is it possible to add the building blocks from one cube to another cube, to form a third, larger cube? In this case a 6 × 6 × 6 cube added to an 8 × 8 × 8 cube does not have quite enough building blocks to form a 9 × 9 × 9 cube. There are 216 (63) building blocks in the first cube, and 512 (83) in the second. The total is 728 building blocks, which is 1 short of 93.
With the original ‘squared’ equation, the challenge was to rearrange the tiles in two squares to form a third, larger square. The ‘cubed’ version of the challenge is to rearrange two cubes made of building blocks, to form a third, larger cube. Apparently, no matter what cubes are chosen to begin with, when they are combined the result is either a complete cube with some extra blocks left over, or an incomplete cube. The nearest that anyone has come to a perfect rearrangement is one in which there is one building block too many or too few. For example, if we begin with the cubes 63 (x3) and 83 (y3) and rearrange the building blocks, then we are only one short of making a complete 9 × 9 × 9 cube, as shown in Figure 4.
Finding three numbers which fit the cubed equation perfectly seems to be impossible. That is to say, there appear to be no whole number solutions to the equation
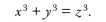
Furthermore, if the power is changed from 3 (cubed) to any higher number n (i.e. 4, 5, 6, …), then finding a solution still seems to be impossible. There appear to be no whole number solutions to the more general equation
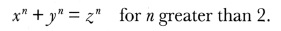
By merely changing the 2 in Pythagoras’ equation to any higher number, finding whole number solutions turns from being relatively simple to being mind-bogglingly difficult. In fact, the great seventeenth-century Frenchman Pierre de Fermat made the astonishing claim that the reason why nobody could find any solutions was that no solutions existed.
Fermat was one of the most brilliant and intriguing mathematicians in history. He could not have checked the infinity of numbers, but he was absolutely sure that no combination existed which would fit the equation perfectly because his claim was based on proof. Like Pythagoras, who did not have to check every triangle to demonstrate the validity of his theorem, Fermat did not have to check every number to show the validity of his theorem. Fermat’s Last Theorem, as it is known, stated that

has no whole number solutions for n greater than 2.
As Wiles read each chapter of Bell’s book, he learnt how Fermat had become fascinated by Pythagoras’ work and had eventually come to study the perverted form of Pythagoras’ equation. He then read how Fermat had claimed that even if all the mathematicians in the world spent eternity looking for a solution to the equation they would fail to find one. He must have eagerly turned the pages, relishing the thought of examining the proof of Fermat’s Last Theorem. However, the proof was not there. It was not anywhere. Bell ended the book by stating that the proof had been lost long ago. There was no hint of what it might have been, no clues as to the proof’s construction or derivation. Wiles found himself puzzled, infuriated and intrigued. He was in good company.
For over 300 years many of the greatest mathematicians had tried to rediscover Fermat’s lost proof and failed. As each generation failed, the next became even more frustrated and determined. In 1742, almost a century after Fermat’s death, the Swiss mathematician Leonhard Euler asked his friend Clêrot to search Fermat’s house in case some vital scrap of paper still remained. No clues were ever found as to what Fermat’s proof might have been. In Chapter 2 we shall find out more about the mysterious Pierre de Fermat and how his theorem came to be lost, but for the time being it is enough to know that Fermat’s Last Theorem, a problem that had captivated mathematicians for centuries, had captured the imagination of the young Andrew Wiles.
Sat in Milton Road Library was a ten-year-old boy staring at the most infamous problem in mathematics. Usually half the difficulty in a mathematics problem is understanding the question, but in this case it was simple – prove that xn + yn = zn has no whole number solutions for n greater than 2. Andrew was not daunted by the knowledge that the most brilliant minds on the planet had failed to rediscover the proof. He immediately set to work using all his textbook techniques to try and recreate the proof. Perhaps he could find something that everyone else, except Fermat, had overlooked. He dreamed he could shock the world.
Thirty years later Andrew Wiles was ready. Standing in the auditorium of the Isaac Newton Institute, he scribbled on the board and then, struggling to contain his glee, stared at his audience. The lecture was reaching its climax and the audience knew it. One or two of them had smuggled cameras into the lecture room and flashes peppered his concluding remarks.
With the chalk in his hand he turned to the board for the last time. The final few lines of logic completed the proof. For the first time in over three centuries Fermat’s challenge had been met. A few more cameras flashed to capture the historic moment. Wiles wrote up the statement of Fermat’s Last Theorem, turned towards the audience, and said modestly: ‘I think I’ll stop here.’
Two hundred mathematicians clapped and cheered in celebration. Even those who had anticipated the result grinned in disbelief. After three decades Andrew Wiles believed he had achieved his dream, and after seven years of isolation he could reveal his secret calculation. However, while euphoria filled the Newton Institute tragedy was about to strike. As Wiles was enjoying the moment, he, along with everyone else in the room, was oblivious of the horrors to come.
2 The Riddler
‘Do you know,’ the Devil confided, ‘not even the best mathematicians on other planets – all far ahead of yours – have solved it? Why, there’s a chap on Saturn – he looks something like a mushroom on stilts – who solves partial differential equations mentally; and even he’s given up.’
Arthur Porges, ‘The Devil and Simon Flagg’
Pierre de Fermat was born on 20 August 1601 in the town of Beaumont-de-Lomagne in south-west France. Fermat’s father, Dominique Fermat, was a wealthy leather merchant, and so Pierre was fortunate enough to enjoy a privileged education at the Franciscan monastery of Grandselve, followed by a stint at the University of Toulouse. There is no record of the young Fermat showing any particular brilliance in mathematics.
Pressure from his family steered Fermat towards a career in the civil service, and in 1631 he was appointed conseiller au Parlement de Toulouse, a councillor at the Chamber of Petitions. If locals wanted to petition the King on any matter they first had to convince Fermat or one of his associates of the importance of their request. The councillors provided the vital link between the province and Paris. As well as liaising between the locals and the monarch, the councillors made sure that royal decrees emanating from the capital were implemented back in the regions. Fermat was an efficient civil servant, who by all accounts carried out his duties in a considerate and merciful manner.
Fermat’s additional duties included service in the judiciary and he was senior enough to deal with the most severe cases. An account of his work is given by the English mathematician, Sir Kenelm Digby. Digby had requested to see Fermat, but in a letter to a mutual colleague, John Wallis, he reveals that the Frenchman had been occupied with pressing judicial matters, thus excluding the possibility of a meeting:
It is true that I had exactly hit the date of the displacement of the judges of Castres to Toulouse, where he [Fermat] is the Supreme Judge to the Sovereign Court of Parliament; and since then he has been occupied with capital cases of great importance, in which he has finished by imposing a sentence that has made a great stir; it concerned the condemnation of a priest, who had abused his functions, to be burned at the stake. This affair has just finished and the execution has followed.
Fermat corresponded regularly with Digby and Wallis. Later we will see that the letters were often less than friendly, but they provide vital insights into Fermat’s daily life, including his academic work.
Fermat rose rapidly within the ranks of the civil service and became a member of the social élite, entitling him to use de as part of his name. His promotion was not necessarily the result of ambition, but rather a matter of health. The plague was raging throughout Europe and those who survived were elevated to fill the places of those who died. Even Fermat suffered a serious bout of plague in 1652, and was so ill that his friend Bernard Medon announced his death to several colleagues. Soon after he corrected himself in a report to the Dutchman Nicholas Heinsius:
I informed you earlier of the death of Fermat. He is still alive, and we no longer fear for his health, even though we had counted him among the dead a short time ago. The plague no longer rages among us.
In addition to the health risks of seventeenth-century France, Fermat had to survive the political dangers. His appointment to the Parliament of Toulouse came just three years after Cardinal Richelieu was promoted to first minister of France. This was an era of plotting and intrigue, and everyone involved in the running of the state, even at local government level, had to take care not to become embroiled in the machinations of the Cardinal. Fermat adopted the strategy of performing duties efficiently without drawing attention to himself. He had no great political ambition, and did his best to avoid the rough and tumble of parliament. Instead he devoted all his spare energy to mathematics and, when not sentencing priests to be burnt at the stake, Fermat dedicated himself to his hobby. Fermat was a true amateur academic, a man whom E.T. Bell called the ‘Prince of Amateurs’. But so great were his talents that when Julian Coolidge wrote Mathematics of Great Amateurs, he excluded Fermat on the grounds that he was ‘so really great that he should count as a professional’.
At the start of the seventeenth century, mathematics was still recovering from the Dark Ages and was not a highly regarded subject. Similarly mathematicians were not treated with great respect and most of them had to fund their own studies. For example, Galileo was unable to study mathematics at the University of Pisa and was forced to seek private tuition. Indeed, the only institute in Europe to actively encourage mathematicians was Oxford University which had established the Savilian Chair of Geometry in 1619. It is true to say that most seventeenth-century mathematicians were amateurs, but Fermat was an extreme case. Living far from Paris he was isolated from the small community of mathematicians that did exist, which included such figures as Pascal, Gassendi, Roberval, Beaugrand and most notably Father Marin Mersenne.
Father Mersenne made only minor contributions to number theory and yet he played a role in seventeenth-century mathematics which was arguably more important than any of his more esteemed colleagues. After joining the order of Minims in 1611, Mersenne studied mathematics and then taught the subject to other monks and to nuns at the Minim convent at Nevers. Eight years later he moved to Paris to join the Minims de l’Annociade, close to the Place Royale, a natural gathering place for intellectuals. Inevitably Mersenne met the other mathematicians of Paris, but he was saddened by their reluctance to talk to him or to each other.
The secretive nature of the Parisian mathematicians was a tradition which had been passed down from the cossists of the sixteenth century. The cossists were experts in calculations of all kinds and were employed by merchants and businessmen to solve complex accounting problems. Their name derives from the Italian word cosa, meaning ‘thing’, because they used symbols to represent an unknown quantity, similar to the way mathematicians use x today. All professional problem-solvers of this era invented their own clever methods for performing calculations and would do their utmost to keep these methods secret in order to maintain their reputation as the only person capable of solving a particular problem. On one exceptional occasion Niccolò Tartaglia, who had found a method for quickly solving cubic equations, revealed his discovery to Girolamo Cardano and swore him to absolute secrecy. Ten years later Cardano broke his promise and published Tartaglia’s method in his Ars Magna, an act which Tartaglia would never forgive. He broke off all relations with Cardano and a bitter public dispute ensued, which only served to further encourage other mathematicians to guard their secrets. The secretive nature of mathematicians continued right up until the end of the nineteenth century, and as we shall see later there are even examples of secret geniuses working in the twentieth century.
When Father Mersenne arrived in Paris he was determined to fight against the ethos of secrecy and tried to encourage mathematicians to exchange their ideas and build upon each other’s work. The monk arranged regular meetings and his group later formed the core of the French Academy. When anyone refused to attend, Mersenne would pass on to the group whatever he could by revealing letters and papers – even if they had been sent to him in confidence. It was not ethical behaviour for a man of the cloth, but he justified it on the grounds that the exchange of information would benefit mathematics and mankind. These acts of indiscretion naturally caused bitter arguments between the well-meaning monk and the taciturn prima donnas, and eventually destroyed Mersenne’s relationship with Descartes which had lasted since the two men had studied together at the Jesuit College of La Flèche. Mersenne had revealed philosophical writings by Descartes which were liable to offend the Church, but to his credit he did defend Descartes against theological attacks, as in fact he had done earlier in the case of Galileo. In an era dominated by religion and magic Mersenne stood up for rational thought.
Mersenne travelled throughout France and further afield, spreading news of the latest discoveries. In his travels he would make a point of meeting up with Pierre de Fermat and, indeed, seems to have been Fermat’s only regular contact with other mathematicians. Mersenne’s influence on this Prince of Amateurs must have been second only to the Arithmetica, a mathematical treatise handed down from the ancient Greeks which was Fermat’s constant companion. Even when he was unable to travel Mersenne would maintain his relationship with Fermat and others by writing prolifically. After Mersenne’s death his room was found stacked with letters written by seventy-eight different correspondents.
Despite the encouragement of Father Mersenne, Fermat steadfastly refused to reveal his proofs. Publication and recognition meant nothing to him and he was satisfied with the simple pleasure of being able to create new theorems undisturbed. However, the shy and retiring genius did have a mischievous streak, which, when combined with his secrecy, meant that when he did sometimes communicate with other mathematicians it was only to tease them. He would write letters stating his most recent theorem without providing the accompanying proof. Then he would challenge his contemporaries to find the proof. The fact that he would never reveal his own proofs caused a great deal of frustration. Rene Descartes called Fermat a ‘braggart’ and the Englishman John Wallis referred to him as ‘That damned Frenchman’. Unfortunately for the English, Fermat took particular pleasure in toying with his cousins across the Channel.
As well as having the satisfaction of annoying his colleagues, Fermat’s habit of stating a problem but hiding its solution did have more practical motivations. First, it meant that he did not have to waste time fully fleshing out his methods; instead he could rapidly proceed to his next conquest. Furthermore, he did not have to suffer jealous nit-picking. Once published, proofs would be examined and argued over by everyone and anyone who knew anything about the subject. When Blaise Pascal pressed him to publish some of his work, the recluse replied: ‘Whatever of my work is judged worthy of publication, I do not want my name to appear there.’ Fermat was the secretive genius who sacrificed fame in order not to be distracted by petty questions from his critics.
This exchange of letters with Pascal, the only occasion when Fermat discussed ideas with anyone but Mersenne, concerned the creation of an entirely new branch of mathematics – probability theory. The mathematical hermit was introduced to the subject by Pascal, and so, despite his desire for isolation, he felt obliged to maintain a dialogue. Together Fermat and Pascal would discover the first proofs and cast-iron certainties in probability theory, a subject which is inherently uncertain. Pascal’s interest in the subject had been sparked by a professional Parisian gambler, Antoine Gombaud, the Chevalier de Méré, who had posed a problem which concerned a game of chance called points. The game involves winning points on the roll of a dice, and whichever player is the first to earn a certain number of points is the winner and takes the prize money.
Gombaud had been involved in a game of points with a fellow-gambler when they were forced to abandon the game half-way through, owing to a pressing engagement. The problem then arose as to what to do with the prize money. The simple solution would have been to have given all the money to the competitor with the most points, but Gombaud asked Pascal if there was a fairer way to divide the money. Pascal was asked to calculate the probability of each player winning had the game continued and assuming that both players would have had an equal chance of winning subsequent points. The prize money could then be split according to these calculated probabilities.
Prior to the seventeenth century the laws of probability were defined by the intuition and experience of gamblers, but Pascal entered into an exchange of letters with Fermat with the aim of discovering the mathematical rules which more accurately describe the laws of chance. Three centuries later Bertrand Russell would comment on this apparent oxymoron: ‘How dare we speak of the laws of chance? Is not chance the antithesis of all law?’
The Frenchmen analysed Gombaud’s question and soon realised that it was a relatively trivial problem which could be solved by rigorously defining all the potential outcomes of the game and assigning an individual probability to each one. Both Pascal and Fermat were capable of independently solving Gombaud’s problem, but their collaboration speeded up the discovery of a solution and led them to a deeper exploration of other more subtle and sophisticated questions related to probability.
Probability problems are sometimes controversial because the mathematical answer, the true answer, is often contrary to what intuition might suggest. This failure of intuition is perhaps surprising because ‘survival of the fittest’ ought to provide a strong evolutionary pressure in favour of a brain naturally capable of analysing questions of probability. You can imagine our ancestors stalking a young deer, and weighing up whether or not to attack. What is the risk that a stag is nearby ready to defend its offspring and injure its assailant? On the other hand what is the chance that a better opportunity for a meal might arise if this one is judged too risky? A talent for analysing probability should be part of our genetic makeup and yet often our intuition misleads us.
One of the most counterintuitive probability problems concerns the likelihood of sharing birthdays. Imagine a football pitch with 23 people on it, the players and the referee. What is the probability that any two of those 23 people share the same birthday? With 23 people and 365 birthdays to chose from, it would seem highly unlikely that anybody would share the same birthday. If asked to put a figure on it most people would guess a probability of perhaps 10% at most. In fact, the actual answer is just over 50% – that is to say, on the balance of probability, it is more likely than not that two people on the pitch will share the same birthday.
The reason for this high probability is that what matters more than the number of people is the number of ways people can be paired. When we look for a shared birthday, we need to look at pairs of people not individuals. Whereas there are only 23 people on the pitch, there are 253 pairs of people. For example, the first person can be paired with any of the other 22 people giving 22 pairings to start with. Then, the second person can be paired with any of the remaining 21 people (we have already counted the second person paired with the first person so the number of possible pairings is reduced by one), giving an additional 21 pairings. Then, the third person can be paired with any of the remaining 20 people, giving an additional 20 pairings, and so on until we reach a total of 253 pairs.
The fact that the probability of a shared birthday within a group of 23 people is more than 50% seems intuitively wrong, and yet it is mathematically undeniable. Strange probabilities such as this are exactly what bookmakers and gamblers rely on in order to exploit the unwary. The next time you are at a party with more than 23 people you might want to make a wager that two people in the room will share a birthday. Please note that with a group of 23 people the probability is only slightly more than 50%, but the probability rapidly rises as the group increases in size. Hence, with a party of 30 people it is certainly worth betting that two of them will share the same birthday.







