Fermat's Last Theorem (Conditions and decisions)

From (8) it follows that for odd a ≠ 1 the numbers cn and bn are also integers, and the identity always holds:

cn/2 – bn/2 = 1 (9)

that for simultaneously integer b, c and n is feasible only for n/2 ≤ 1, n = 1, 2, which is what needed to be proved.

The proof can be carried out in a slightly different way. All numbers of the equality an + bn = cn, where a ≠ 1, b and n are arbitrarily chosen natural numbers, c is a real positive number, through transformations (1)…(4) can be expressed as terms of identity (5).

Let us take the factor aq ≠ 0 out of brackets and divide all terms of identity (5) by it:

(ak +1)/2)2 – (ak – 1)/2)2 = ak (10) where k = p – q.

In accordance with the properties of the exponential function, arbitrarily chosen natural numbers a, b and n, for example, from equality (5), correspond to a single value k satisfying the condition:

bn = (ak – 1)/2)2 (11) then cn = bn + ak = (ak + 1)/2)2, or cn – bn = ak (12) where a, b and n are integers.

From (10), (11) and (12) it follows: : cn/ 2 – b n/2 = 1 (13) that is, the numbers b and c can be simultaneously integers only when n/2 ≤ 1, or n = 1, 2 . For n = 2, b and c are consecutive integers. Euclid also proved that any odd number can be expressed as the difference of the squares of two consecutive integers, which can be found using identity (10) for any integer k and odd a ≠ 1.

Note that equality (12) was obtained by dividing equality (5) by the factor a 2q, while the number bn in these equalities is the same, which 2q = 1, q = 0 , p = k = n, and the identity (10) takes the form of identity (8).

Note also that identities (8) and (10) are valid not only for integer values of a. By substituting any rational fraction for a and setting k = n = 2, you can find all Pythagorean numbers.

The above transformations of the Fermat equality over the set of natural numbers show that with the help of a finite number of arithmetic operations it is always reduced to identity (13), which proves the theorem.

Proof of Fermat's theorem using elementary algebra methods

Later, Bobrov A.V. published another proof of Fermat’s Theorem.

Fermat's theorem states that the equality an + bn = cn for natural a, b, n can only hold for integers n ≤ 2.

Consider the equality C – B = A, (1) where A, B, C are coprime natural numbers, that is, numbers that do not have common integer factors other than 1. In this case, two numbers are always odd. Let –A = an – an odd number, a ≠ 1 and n – natural numbers. For any real positive number, we can perform the operation of finding the arithmetic value of the root, that is, equality (1) can be written as:

(√C + √B) (√C –√B) = A1 A2 = A, (2) where A1 = √C+√B и A2 =√C-√B -

real positive factors of a number In accordance with the properties of the exponential function, for any of the real positive numbers A1 and A2 there are unique values of the numbers 0< q< p < ∞ satisfying the equalities A1 = a p A2 = a q, (3)

From equalities (2) and (3) it follows:

√C = (ap + aq)/2, √B = (ap – aq)/2, A = a p+q = a n (4)

Since p > q, always takes place p-q=k,, or аp= аk × аq, that is, the numbers A,B,C contain a common factor, which contradicts the condition of their mutual you just. This condition is fulfilled only when aq = 1, that is, when q= 0, p = n.

Then equalities (4) take the form:

√C = (an + 1)/2, √B = (an – 1)/2, A = a n (5)

whence follows

√C - √B = 1 (6)

that is, for coprime A,B,C, the numbers c =√C, b = √B are always two consecutive integers. Euclid also proved that every odd number is expressed as the difference of the squares of two consecutive integers, that is, equality (1) for natural coprime a, b, c can only be expressed as the equality c2 -b2 = a2. (7)

The validity of the above proof can be illustrated by the following example.

Let the numbers a,b,c in Fermat’s equality be coprime integers, and let n be even. Then the numbers cn/2, bn/2, their sum A1 = ap and difference A2 = aq are also integers, the exponent p>q.

Integers cn/2 = ( ak+q + aq)/2, bn/2 = (ak+q – aq)/2 are coprime if they do not contain common integer factors other than 1. This condition is satisfied only if the common integer factor is aq = 1, that is, q = 0, p = n.

Then the difference cn/2 - bn/2 = 1, which for simultaneously integer b,c,n can only occur when n/2 ≤ 1, that is, when n = 1, 2.

Second proof 2001-2004

In 2001-2004, V. A. Gorbunov published a simple proof of Fermat’s last theorem at MSTU.

Proof of Fermat's Last Theorem

Fermat's theorem states that there are no integer solutions to the equation

xn + yn = zn (1) for any natural number n > 2 (xyz ≠0).

Proof

By introducing the transformations u = x/z, v = y/z, (2) equation (1) is reduced to the form un + v n = 1. (3)

It is obvious that the variables u and v satisfy the inequalities 0 ≤ u ≤ 1 and 0 ≤ v ≤ 1. (4)

Let us denote the sum un + vn by Sn , and call the graph of the equation Sn = 1 the Fermat curve of the n-order. Figure 1 shows a square containing: a unit circle S2 =1 and a Fermat curve Sn =1 (n ≥ 3). Due to the symmetry of equation (3) with respect to the variables u and v, in what follows we will consider only the upper part of the square with respect to the secondary diagonal (matrix terminology). For any natural number z (z > 1), we apply a uniform grid on the unit square with a rational step h = z/2, Figure 1. The larger z, the smaller h, that is, the denser the grid in the unit square. The statement of Fermat's theorem is equivalent to the fact that whatever the number z (and, consequently, h), Fermat curves do not pass through the nodal (rational) points in the unit square, which is what remains to be proved.

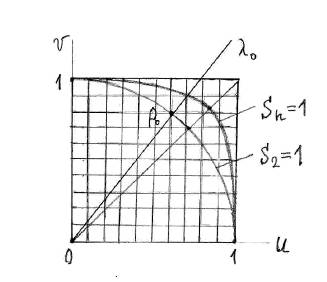
Fig.1. Unit circle S2 =1 and Fermat curve Sn = 1 (n =3,4,…) in a unit square covered with a uniform grid with a step h =1/z.

It is known that there is an infinite number of rational points on the unit circle. If, when dividing a unit square for a natural number z0, the rational point of the unit circle P0 (x0/z0, y0/z0) turns out to be a nodal point on the mesh, then we will call this point Pythagorean, and the ray passing through it (coming from the origin) will be called Pythagorean beam ƛ0.

If the Pythagorean number z0 is prime, then at the top of the unit square on the unit circle there will be one Pythagorean point on the grid for z0.

1. Any point in the unit square M0 (x0/z1, y0/z2) with rational coordinates can become a node on the grid for a natural number z = z1 * z2, if NOD (z1 * z2) = 1= 1. Thus, in what follows about a rational point, we will assume that the denominators of the coordinates of the point are the same.

For composite numbers of the form z = k * p1* p2 *…*pl,, where p1 p2 ,…, pl are prime numbers of the form 4n+1, and k is a natural number whose factorization does not contain Pythagorean numbers, then in the upper part of the unit square there will be l2 Pythagorean points on the unit circle for a grid with a step h = 1/ z.

Let us denote by ƛ the ray forming an angle ƛ with the Ou axis. In addition to the designation, we will give a numerical value to the parameter ƛ/ƛ = tgȹ. Then the coordinates of the points on this ray are related by the relation v = ƛ*u. Substituting instead of v into equation (3) ƛ*u, we obtain the coordinates of the point Фn lying at the intersection of the ray ƛ with the Fermat curve Sn =1:

un = 1/ n√(1+ ƛ n), vn = ƛ / n√(1+ ƛ n) (5)

In what follows, we will be interested only in rays passing through nodal (rational) grid points in a unit square. On such rays the parameter ƛ takes rational values. We will distinguish between rays ƛ 0 passing through rational points on the unit circle and rays ƛ* passing through rational points in the unit square and not lying (points) on the unit circle.

Let us prove a lemma that will be useful to us later.

Lemma.

1). If the rational point Ф* ( x*/z0, y*/z0) lies on the ray ƛ 0, then the length of the radius vector R* of this point is a rational number, (x*, y* are natural numbers).

2). If the rational point Ф* ( x*/z, y*/z) lies on the ray ƛ*, then the length of the radius vector R* is an irrational number (x*, y*, z* are natural numbers).

Let us prove the first part of the lemma.

Let P0 (x0/z0, y0/z0) be a point with rational coordinates that belongs to the unit circle (P0 ϵ S2 =1). Then the coordinates of this point satisfy the equation x20 + y20 = z20, (6) where x0, y0, z0 are natural numbers. Let the point Ф* ( x*/z0, y*/z0) with rational coordinates lie on the ray ƛ 0 passing through the point P0, (Ф* ϵ S2 =1).

Then ƛ 0 = y0/ x0 = y*/ x* and the length of the radius vector of point Ф* will be equal to R* = √((x*/z0)2 + (y*/z0)2) = x*/ z0 √(1+ ƛ 02) = x*/ z0 √(1+(y0/x0)2) = x*/ x0 – rational number.

2. The proof of this formula is given in Appendix 2.

Let us prove the second part of the lemma.

Let the point Ф*(x*/z*, y*/z*) with rational coordinates lie on the ray ƛ * which intersects the unit circle at the irrational point P* (u* ,v*), u*= m/k*, v* = n/k*.. Since ƛ * = y*/x* is a rational number, then the numerators of the fractions u* , v* are rational numbers: ƛ * = n/m. Whence it follows that the denominator k* = √(m2+n2) is an irrational number. The length of the radius vector of point Ф* will be equal to

R* = √( (x*/z*)2 + (y*/z*)2) = x*/z* √(1+(y*/x*)2) = x*/z* √(1+(n/m)2) = x*k*/z*m is an irrational number.

Thus, the length of the radius vector of a rational point on the ray ƛ0 (passing through the rational point of the unit circle) is a rational number, and the length of the radius vector of a rational point lying on the ray ƛ* (intersecting the unit circle at the irrational point) is the number irrational.Докажем вторую часть леммы.

Let x0, y0, z0 be a Pythagorean triple of numbers with pairwise coprime numbers. Such Pythagorean triplets of numbers are called primitive. Then on the grid in the unit square for the natural number z0 on the unit circle there is a Pythagorean point P0 (u0, v0), where u0 = x0/ z0, v0 = y0/ z0. The ray ƛ0 passing through the point P0 does not contain other grid nodes for z0. Let's prove it.

Let the nodal point Ф*(x*/z0, y*/z0) of the grid for z0 lie on the ray ƛ 0. Then y* = ƛ 0 x* = (y0/x0) x* (7)

Since x0 and y0 are relatively prime numbers, then y* will be an integer if x* is a multiple of x0, that is, x x* = m x0 where m is a natural number, m > 1. Then y* = my0 the coordinates of the point Ф* will be: u* = m x0 / z0, v* = m y0 / z0. It follows that the value of the radius vector of the point Ф* will be R* = m, where m is an integer. Since the largest value of the length of the radius vector of any point inside the unit square is less than √2, the point will be outside the unit square.

So, if the Pythagorean triple of numbers ( x0 , y0 , z0 ) is primitive, then on the Pythagorean ray ƛ0, except for the Pythagorean point P0 on the unit circle, there are no other nodal points of the grid. Then on the grid in the unit square for a natural number z0, all Fermat curves intersect the ray ƛ0 not at nodal points and, therefore, according to equation (1), there will be no integer solutions at these points. Then the transformed equation (3) will have irrational solutions for all n >2:

un = 1 /n√(1+ ƛ 0n ) = x0 / n√( x0n +y0n ), vn = ƛ 0 /n√(1+ ƛ 0n ) = y0 / n√( x0n +y0n )

Note that according to formula (8), the coordinates of the points of the Fermat curves on the ray ƛ 0 are formed from the coordinates of the Pythagorean point P0 (x0 / z0, y0 / z0) and, therefore, do not depend on other partitions of the unit square (obviously, the coordinates of the points do not depend at all on the partition of the unit square).

A comment.

On the one hand, the lemma states that on the Pythagorean ray ƛ 0 the length of the radius vector of the point Ф* with rational coordinates R* is a rational number. On the other hand, on a grid for z0 on which the point P0 on the unit circle is a nodal point (that is, the unit square for a given partition is integerly decomposed over the cells of the partition into the sum of two squares), there are no other nodal points on the ray ƛ 0 and we draw the conclusion (which has yet to be formally proven) that all Fermat curves intersect the Pythagorean ray ƛ 0 at irrational points. Let's give an example.

Example 1.

z0 = 5, P0 (3/5 , 4/5), ƛ 0 = 4/3. With such a partition (partition step h =1/5), the unit square can be decomposed into the sum of two squares with sides 3h and 4h. It is obvious that there is an infinite number of rational points on the ray, for example Ф* (1/2, 2/3). On a grid in a unit square for z = 6, this point will be a nodal point and lie on the ray ƛ0, Ф* (3/6, 4/6). The radius vector of this point is a rational number, R* =5/6. If we combine these two grids, that is, cover a unit square with a grid z1 = z0 , z = 30, then both points P0 (18/30, 24/30) and Ф* (20/30, 15/30) will be nodal points on this grid and are on the same ray ƛ0. The partitioning step of this grid is h = 1/30 and point P0 (18/30, 24/30) still splits the unit square into the sum of two squares with sides 18h and 24h.

In other words, let us make the assumption that some rational point Ф*n lies at the intersection of the Fermat curve Sn = 1 with the Pythagorean ray ƛ0, then we can always select a grid in the unit square in which both P0 and Ф*n will be nodal at the same time.

Let's return to the proof of the theorem. Since the numerators of the coordinates of the point Ф*n (un ,vn), (formulas (8)), are natural numbers, then solutions (8) will be irrational if we prove that n√ ( xn0+yn0) for n > 2 is an irrational number . Let's prove it.

Let for some natural number n, (n > 2 ), n√ ( xn0+yn0) = z10 /k – a rational number, where z10 and k are coprime natural numbers and the point Ф*n (kx0 / z10 , ky0 / z10 ) lies at the intersection of the Fermat curve Sn = 1 with the Pythagorean ray ƛ0. It is obvious that z10 /k < z0, since the length of the radius vector of the point Фn is greater than one, (Fig. 1)

Rn = √( (kx0 / z10 )2 + (ky0 / z10)2) = kz0 / z10 > 1 (9)

From the assumption that the point Ф n with rational coordinates lies on the Fermat curve Sn = 1, it follows that equation (1) must have an integer solution (kx0)n + (ky0)n = (z10)n (10)

From the last equation it follows that z10 must be divisible by k. But, by assumption, z10 and k are relatively prime numbers. Therefore, n√( (xn0 + yn0 ) cannot be a fractional rational number.

Let now n√(xn0+ yn0)be a natural number and z10 –z0. Then on the grid in the unit square for the natural number z10, the point Фn (xn0/ z10, y0/ z10) is a nodal point and lies on the Fermat curve Sn = 1, according to the proposal.

Obviously, for Pythagorean triples of numbers (x0, y0, z0), for which z0 = y0 +1 (for example, (3,4,5), (5,12,13), etc.), n√(xn0+ yn0) cannot be an integer, since in this case the inequalities must be satisfied.

y0 < n√(xn0+ yn0) < y0 +1 (11)

In other cases, when z0 > y0 +1, for example (20, 21, 29) from the assumption that the point Фn (x0/ z10, y0/ z10) lies on the Fermat curve Sn = 1, it follows that this point will be a nodal point on grid in the unit square for a natural number z10 that is not a multiple of z0 , (z0 > z10 and z0 is a prime number). According to formula (8), with n =2, the solution to equation (3) must be rational, and equation (1) must be integer. But on the grid in the unit square for z10, the point P0 will not be a nodal point and, therefore, with n = 2 at this point, equation (1) will not have an integer solution.

Therefore n√(xn0+ yn0) is an irrational number.

Proving that n√(xn0+ yn0) is irrational is not necessary. This follows from the previously proven statement that on the Pythagorean ray ƛ0 on the grid in the unit square for z0, except for the point P0 on the unit circle, there are no other nodal points. This is equivalent to the statement that for a right triangle with legs x0 and y0, the sum of legs to any other power n > 2 will not be a rational number (or rather an integer).

From the irrationality of n√(xn0+ yn0) for n > 2 it follows that the length of the radius vector of the point Фn lying at the intersection of the Fermat curve Sn = 1 with the Pythagorean ray ƛ0 is also an irrational number.

Rn = √(u2n+ vn2) = √( (x0/√(x0n+ y0n))2 + (y0/√(x0n+ y0n))2) = z0/n√(x0n+ y0n) (12)

It is obvious that there are infinitely many rational points on the ray ƛ0 and each of them, with an appropriate choice of the partitioning step of the unit square h + 1/z, can become a node on the grid for a natural number z. According to the lemma, the length of the radius vector of the point Ф* on the ray ƛ0 is a rational number. Consequently, for any partitions of the unit square, on the Pythagorean ray ƛ0 the inequality R* ≠ Rn holds, (13) where Rn is the length of the radius vector of the point Фn of the Fermat curve Sn = 1, and R* is the length of the radius vector of any rational point on the beam ƛ0.

The coordinates of the point Фn on the Pythagorean ray ƛ 0 can be written as

Фn ( x0/n√(x0n+ y0n), y0/n√(x0n+ y0n)) or Фn ( x0 hn), ( y0 hn), where hn = Rn/ z0 = 1/ n√(x0n+ y0n) (14)

The coordinates of the Pythagorean point P0 can be written as P0(x0 h0, y0 h0), where h0 = 1/ z0 is the step of partitioning the unit square. (15)

Obviously, hn > h0,, since Rn > 1, (hn = h0 Rn)

If we construct a square with a side equal to the length of the radius vector of the point Фn and cover this square with a uniform mesh with a step of hn = Rn/z0, then the point Фn in this square will be a nodal (Pythagorean) point. At this point, as well as for the Pythagorean point P0 ϵ S2 = 1, the Pythagorean theorem is fulfilled integer over cells (Fig. 2).

It remains for us to consider the rays ƛ* passing through the nodal (rational) points of various partitions of the unit square, which are not Pythagorean rays for any partitions. These rays intersect the unit circle at irrational points. For example, ƛ*= 3/ 2 or ƛ* = 6/5, etc.

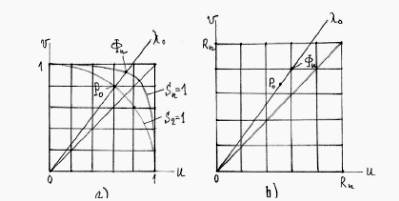
Fig.2. a – uniform grid in the unit square for the Pythagorean number z0, (h0 = 1/ z0)

P0 (x0 / z0, y0 / z0) – Pythagorean point: Фn

Конец ознакомительного фрагмента.

Текст предоставлен ООО «Литрес».

Прочитайте эту книгу целиком, купив полную легальную версию на Литрес.