
Полная версия
All sciences. №3, 2023. International Scientific Journal

All sciences. №3, 2023
International Scientific Journal
Authors: Aliyev Ibratjon, Abduraxmonov Sultonali Mukaramovich, Odilov Sanjar Sadiqjonovich, Toyirov Nurmuxammad Sultonaliyevich, Obidov Foziljon Oripovich, Abdusalyamova Toira
Editor-in-Chief Ibratjon Xatamovich Aliyev
Illustrator Ibratjon Xatamovich Aliyev
Illustrator Obbozjon Xokimovich Qo'ldashov
Illustrator Sultonali Mukaramovich Abduraxmonov
Cover design Ibratjon Xatamovich Aliyev
Cover design Ra'noxon Mukaramovna Aliyeva
Acting scientific supervisor Sultonali Mukaramovich Abduraxmonov
Economic Manager Farruh Murodjonovich Sharofutdinov
Economic consultant Botirali Rustamovich Jalolov
Proofreader Gulnoza Muxtarovna Sobirova
Proogreader Abdurasul Abdusoliyevich Ergashev
Proofreadfer Ekaterina Aleksandrovna Vavilova
© Ibratjon Aliyev, 2023
© Sultonali Mukaramovich Abduraxmonov, 2023
© Sanjar Sadiqjonovich Odilov, 2023
© Nurmuxammad Sultonaliyevich Toyirov, 2023
© Foziljon Oripovich Obidov, 2023
© Toira Abdusalyamova, 2023
ISBN 978-5-0059-9245-1 (т. 3)
ISBN 978-5-0059-5900-3
Created with Ridero smart publishing system
PHYSICAL AND MATHEMATICAL SCIENCES
THE IMPORTANCE OF DIFFERENTIAL EQUATIONS IN THE STUDY OF GENERAL LAWS AND THE SIMPLEST CASES OF TRANSFORMATION
Aliev Ibratjon Khatamovich
2nd year student of the Faculty of Mathematics and Computer Science of Fergana State University
Ferghana State University, Ferghana, Uzbekistan
Аннотация. Изучение окружающего мира непосредственно сводит к необходимости ведения тех или иных прогнозов, которые сводятся уже к важности установления для них основных законов мироздания, которые можно наблюдать в ходе изучения тех или иных явлений. При этом часто использование физических законов, возможные для описания с использованием не только обычных уравнений, но и дифференциальных уравнений, первого и вторых порядков, в том числе и большого количества уравнений в частных производных, довольно часто используемых при этом исследовании и понимании.
Ключевые слова: дифференциальные уравнений в частных производных, обыкновенные дифференциальные уравнения, математическое моделирование, аналогия, закономерности.
Annotation. The study of the surrounding world directly reduces to the need to make certain forecasts, which are already reduced to the importance of establishing for them the basic laws of the universe, which can be observed during the study of certain phenomena. At the same time, there is often the use of physical laws that are possible to describe using not only ordinary equations, but also differential equations of the first and second orders, including a large number of partial differential equations, quite often used in this study and understanding.
Keywords: partial differential equations, ordinary differential equations, mathematical modeling, analogy, regularities.
Coming to the study of the laws of the world in physical science, certain laws were most often distinguished, the initial ones among which are the mechanical laws created by Newton and developed mathematically from his side, along with other scientists, among whom the figure of Leibniz stands out vividly. For an example of this statement, we can give the differential forms of the basic equations of motion (1), which in turn are reduced to certain values in the formulas of acceleration (2), force (3), work (4), power (5) and others.
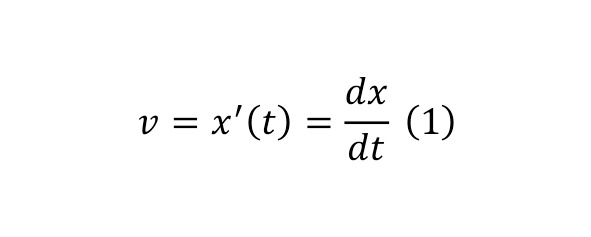
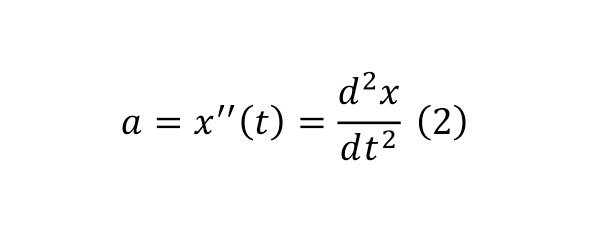
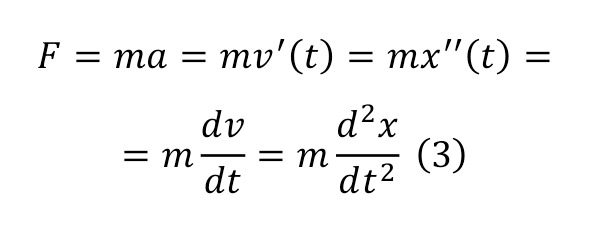
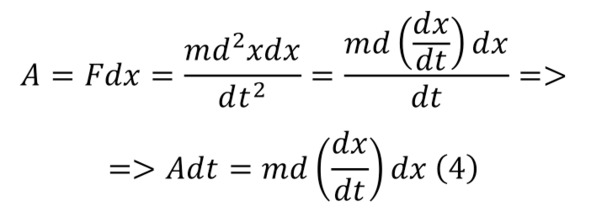
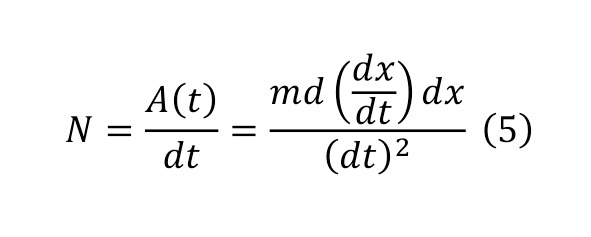
The present moments of understanding can most often be considered precisely in differential forms of meaning, for the reason that they can be numerically determined by introducing some transformations, namely, by converting (6) and taking a certain integral with the establishment of certain boundaries (7).
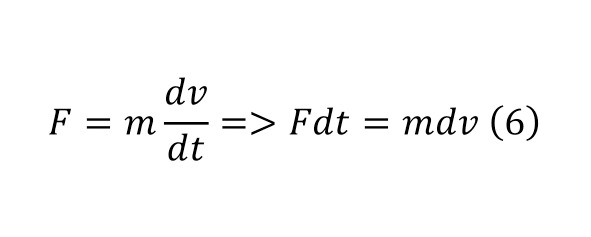
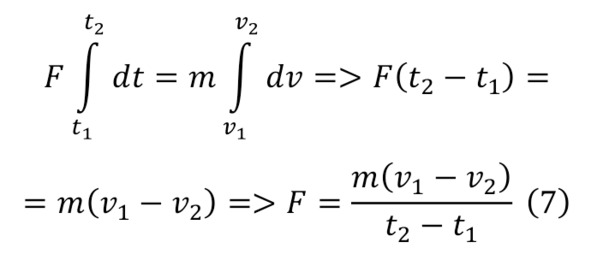
Such directions are developed not only in mechanical terms, but also in other branches of physics, electrostatics, electrodynamics, magnetostatics, magneto-dynamics and others can be a vivid example of this. To prove this, it is enough just to mention that the very concept of current strength is a derivative of the charge time, and voltage is a derivative of the work charge.
This statement can be given for a large number of very different understandings, but the important fact is that such an approach, unlike classical mathematical regulation, becomes the only one when it is necessary to describe the gravitational characteristics of space on the scale of the entire space. An example of this kind of phenomena, where the use of derivatives and, accordingly, differential equations becomes known quantum physics.
However, on the scale of phenomena where the classical mathematical apparatus can no longer perform its functions, it is not so much the usual classical derivatives that are reduced to ordinary differential equations that are important, unless, of course, the simplest cases are not taken into account, a vivid example of which is overcoming the potential well of a particle or describing its motion, or other similar trivial cases, only partial differential equations are more interesting.
Used literature
1. Pontryagin L. S. Ordinary differential equations. – M.: Nauka, 1974.
2. Tikhonov A. N., Samarsky A. A. Equations of mathematical physics. – M.: Nauka, 1972.
3. Tikhonov A. N., Vasilyeva A. B., Sveshnikov A. G. Differential equations. – 4th ed. – Fzimatlit, 2005.
4. Umnov A. E., Umnov E. A. Fundamentals of the theory of differential equations. – Ed. 2nd – 2007. – 240 p.
5. Charles Henry Edwards, David E. Penny. Differential Equations and the problem of eigenvalues: Modeling and calculation using Mathematica, Maple and MATLAB = Differential Equations and Boundary Value Problems: Computing and Modeling. – 3rd ed. – M.: "Williams", 2007.
6. Elsholts L. E. Differential Equations and Calculus of Variations. – M.: Science, 1969.
SOME OPERATIONS AND SPECIAL CASES OF MATHEMATICAL ANALYSIS IN THE EXPONENTIAL SET
Aliev Ibratjon Khatamovich
2nd year student of the Faculty of Mathematics and Computer Science of Fergana State University
Ferghana State University, Ferghana, Uzbekistan
Аннотация. Важность определения и преобразования ингенциальных чисел и настоящего множества с каждым днём становится всё более очевидном, особенно с входом данного понятия в математическую физику, но и как чисто математический объект они представляют не малый интерес, хотя при этом имеют и практическое применение. В настоящей работе, описаны методы проведения некоторых алгебраических операций с ними, в том числе с использованием формулы Эйлера и интеграллами.
Ключевые слова: ингенциальные числа, математический анализ, алгебраические операции, формула Эйлера, интегрирование, производные.
Annotation. The importance of defining and converting exponential numbers and a real set is becoming more and more obvious every day, especially with the entry of this concept into mathematical physics, but as a purely mathematical object they are of no small interest, although they also have practical applications. In this paper, methods of performing some algebraic operations with them are described, including using Euler’s formula and integrals.
Keywords: inertial numbers, mathematical analysis, algebraic operations, Euler formula, integration, derivatives.
The very process of logarithmization of an exponential number of a general form can be seen in (1).
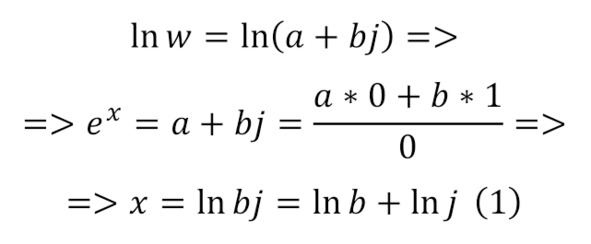
Thus, when logarithming, 2 parts of the expression itself are formed – the real one, as the natural logarithm of the coefficient of the ingential part and the logarithm of the ingential unit, which is defined in (2).

That is, in this case, the question arises to what degree it is necessary to raise the Euler number so that it gives an exponential unit. The answer is quite simple – it is a negative logarithm of zero (2) from this it follows that the logarithm of the exponential number is (3).
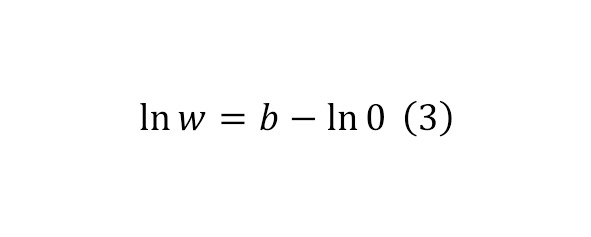
It is also interesting to solve the Euler equation with a tangential unit, and then with a general form of an exponential number, which was described further, taking the expressions as unknowns. And for this, we can initially proceed from Taylor expansions (4—6).
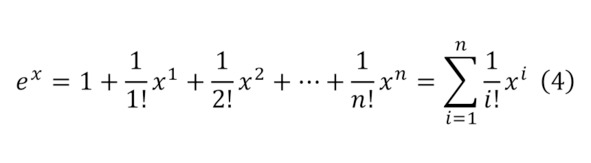
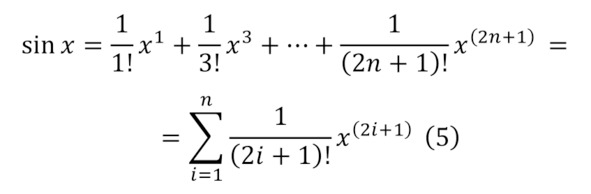
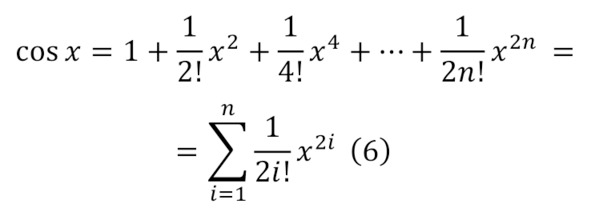
Which is easily proved, since when the unknown is zeroed, the sine in (5) is also zeroed, and the cosine in (6) is equal to one. And it already follows from this (7).
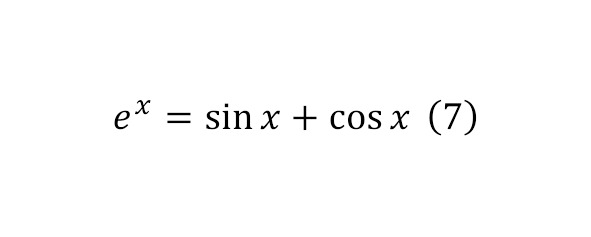
And the unknown in (7) can be all kinds of numbers, both complex, when substituting which the remarkable Euler equality follows, and exponential. And to begin with, let’s consider a special case with an exponential unit and perform the following transformations (8).
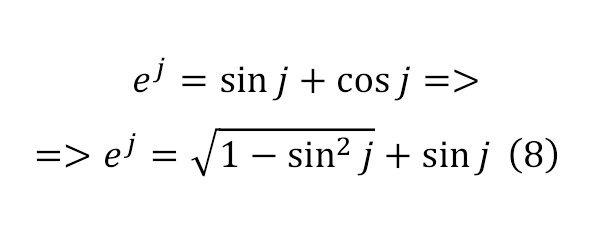
Based on this relation, we perform transformations in (9), leading to equation (10), while taking into account that this expression is identical, it is possible to differentiate both parts of the equation in (11) by performing the corresponding transformations.
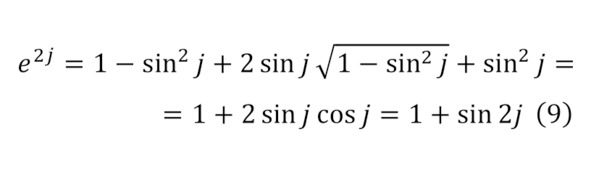
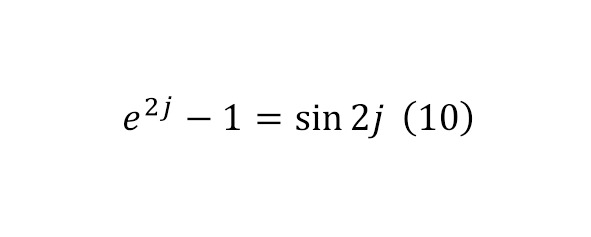
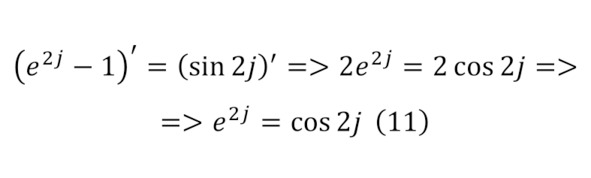
Since the final equality (11) can be represented as in (12), further carrying out additional differentiation, also introducing the condition that this is an identity, and in (13) the differentiation process for the right side of equality is described in detail. And for the left part there is no need for a detailed painting.
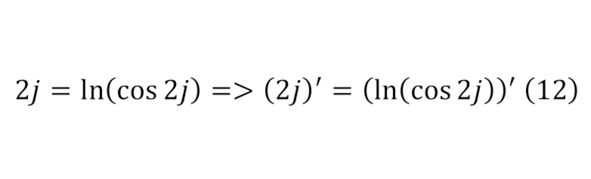
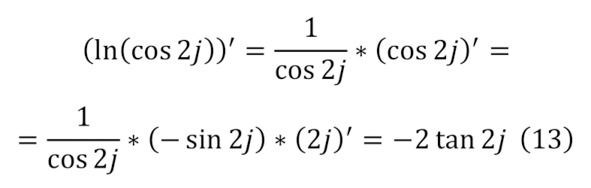
When the differentiation is made, it is enough to make elementary transformations, we get the trigonometric form of the special case (14).
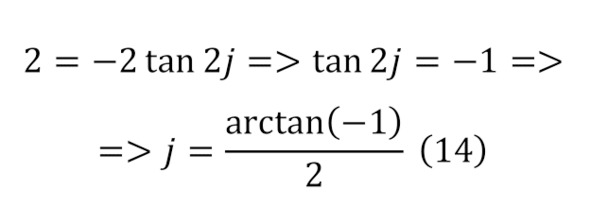
Now, when the general form for the doubly differentiated case is obtained, it is necessary to return to the primordial ones, because this is the identity, resulting in the following equalities (15—16).
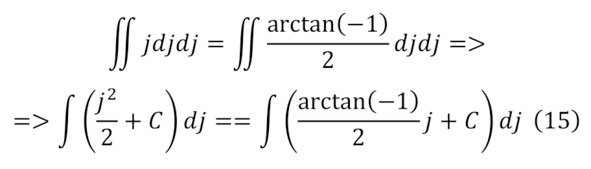
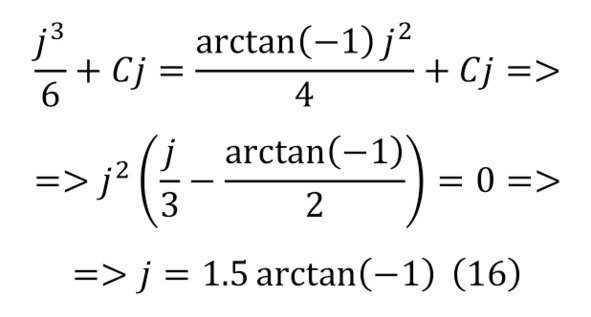
And indeed, this value is close to the most potential value, so this expression can be considered the second kind of writing of the exponential unit. Now, it is possible to proceed to the solution of the Euler equation for the general form of the intentional numbers, having carried out the first substitution and the usual replacement operations at stage (17) and (18) at the beginning.
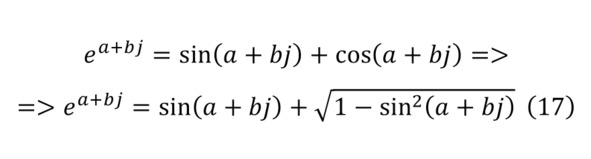
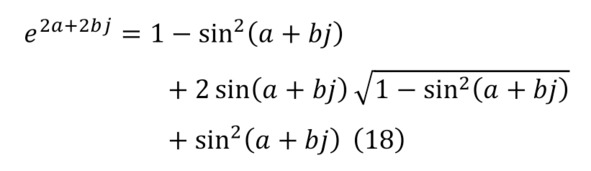
When the necessary transformations come to an end, and other actions no longer take place, it is also sufficient to differentiate both parts of equality as a valid identity (19).
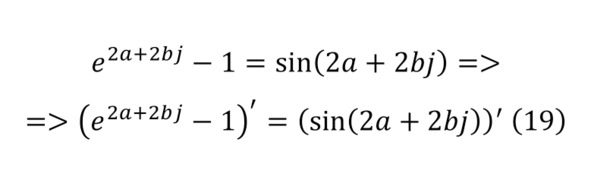
Differentiating the first part of the equality, we can come to the result in (20), and for the second part, the calculations will continue throughout (21).
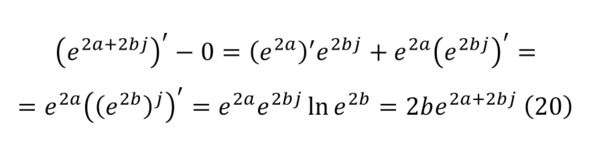
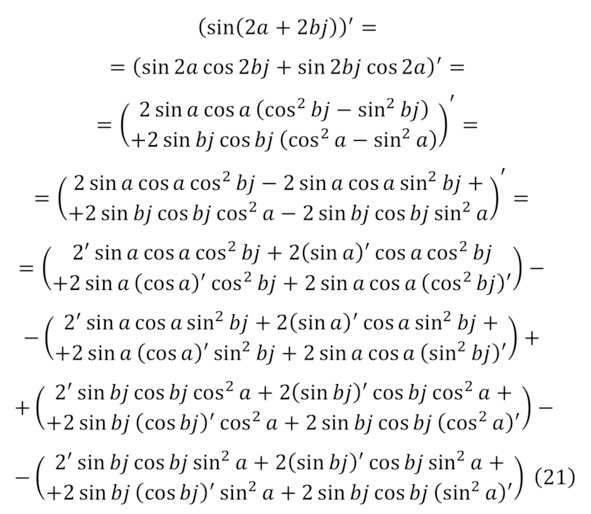
Then, applying (22—25), one can come to the form (26).
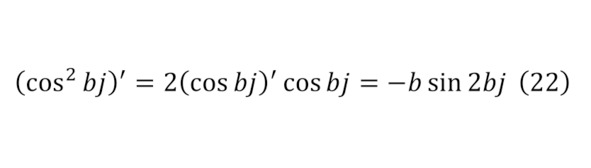

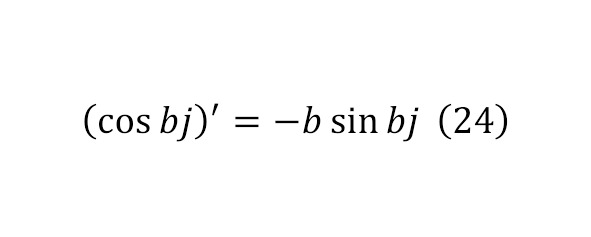
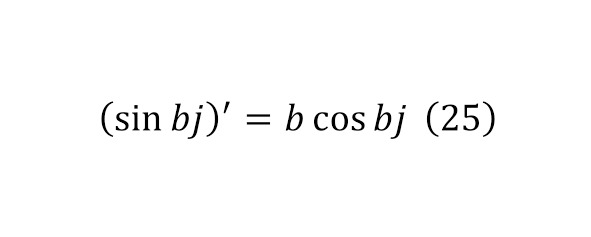
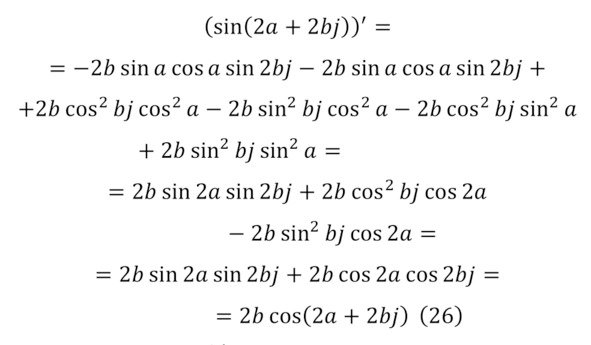
As a result, it is enough to equalize both results in (20) and (26), since these are two parts of the identity, and then get (27) with the necessary simplification, and already in (28) with additional simplification and differentiation as an identity.
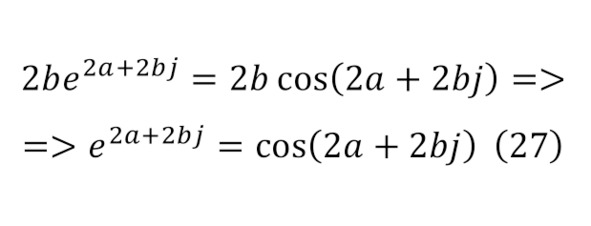
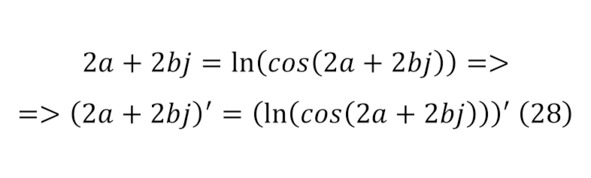
At the same time, the differentiation of the first part of equality is obvious in (29), as well as the second in (30), after which equality and the resulting transformations can be introduced into (31).
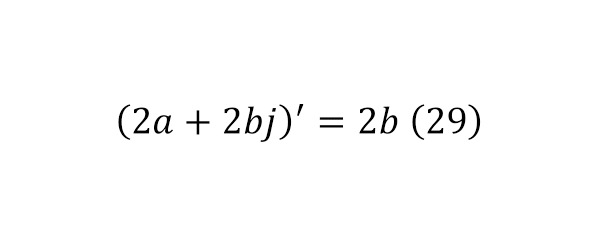
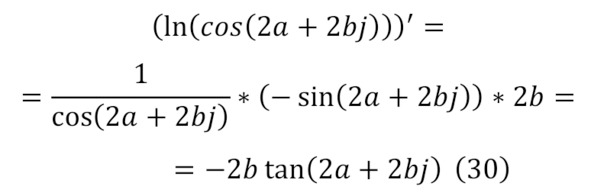
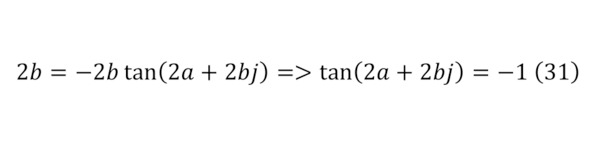
As a result, equalities are formed that need to be integrated twice, because their derivatives were taken earlier, getting (32).
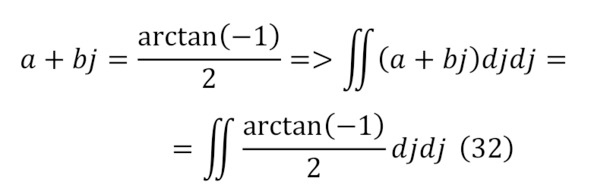
Integrating the first part, a separate result is obtained in (33) and integrating the second part in (34).
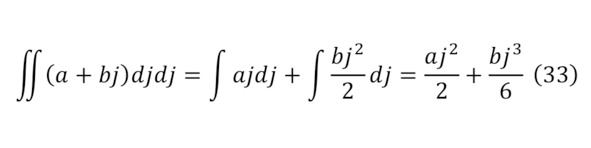

Thus, it is possible to arrive at equality (35), from where it is possible to arrive at another equality in the same equation.

The result is really quite surprising, but this is equality (35), which came out after substituting the general form of an ingential number into Euler’s formula and the solution for this case is the ingential number (36). Thus, this is the first full-fledged equation, the solution of which was an intentional number.
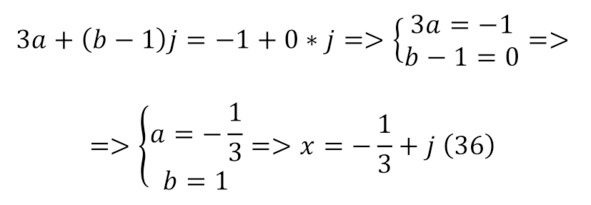
Although the complex numbers themselves are located on the axis of numbers, this interval can also be expressed on the tangential plane. This coordinate system has an axis starting from infinity as the ordinate, and the abscissa has all real numbers. Thus, all exponential numbers can be represented on such a rectangular coordinate system, in the case of adding complex numbers – already in space.
Used literature
1. I. V. Bargatin, B. A. Grishanin, V. N. Zadkov. Entangled quantum states of atomic systems. Editorial office named after Lomonosov. 2001.
2. G. Kane. Modern elementary particle physics. Publishing house Mir. 1990.
3. S. Hawking. The theory of everything. From singularity to infinity: the origin and fate of the universe. Publishing house AST. 2006.
4. S. Hawking, L. Mlodinov. The supreme plan. A physicist's view of the creation of the world. Publishing house AST. 2010.
5. T. D'amour. The world according to Einstein. From relativity theory to string theory. Moscow Publishing House. 2016.
6. S. Hawking, L. Mlodinov. The shortest history of time. Amphora Publishing House. 2011.
ABOUT RESEARCH ON THE COLLATZ HYPOTHESIS IN THE FACE OF A MATHEMATICAL PHENOMENON
Aliev Ibratjon Khatamovich
2nd year student of the Faculty of Mathematics and Computer Science of Fergana State University
Ferghana State University, Ferghana, Uzbekistan
Аннотация. Когда об этой задаче рассказывают молодым математикам – их сразу предупреждают, что не стоит браться за её решение, ибо это кажется невозможным. Простую на вид гипотезу не смогли доказать лучшие умы человечества. Для сравнения, знаменитый математик Пол Эрдеш сказал: «Математика ещё не созрела для таких вопросов». Однако, стоит подробнее изучить данную гипотезу, что и исследуется в настоящей работе.
Ключевые слова: гипотеза Коллатца, числа-градины, ряды, алгоритм, последовательности, доказательства.
Annotation. When young mathematicians are told about this problem, they are immediately warned that it is not worth taking up its solution, because it seems impossible. A simple-looking hypothesis could not be proved by the best minds of mankind. For comparison, the famous mathematician Paul Erdos said: «Mathematics is not yet ripe for such questions.» However, it is worth studying this hypothesis in more detail, which is investigated in this paper.
Keywords: Collatz hypothesis, hailstone numbers, series, algorithm, sequences, proofs.
In short, its essence is as follows. A certain number is selected and if it is not even, it is multiplied by 3 and 1 is added, if it is even, then divided by 2.
We can give an algorithm of this series for the number 7:
7 – 22 – 11 – 34 – 17 – 52 – 26 – 13 – 40 – 20 – 10 – 5 – 16 – 8 – 4 – 2 – 1
Next, a cycle is obtained:
1 – 4 – 2 – 1 etc.
This leads to the hypothesis that if you take any positive integer, if you follow the algorithm, it necessarily falls into the cycle 4, 2, 1. The hypothesis is named after Lothar Collatz, who is believed to have come to this hypothesis in the 30s of the last century, but this problem has many names, it is also known as the Ulam hypothesis, Kakutani's theorem, Toitz's hypothesis, Hass's algorithm, the Sikazuz sequence, or simply as "3n+1".
How did this hypothesis gain such fame? It is worth noting that in the professional environment, the fame of such a hypothesis is very bad, so the very fact that someone is working on this hypothesis may lead to the fact that this researcher will be called crazy or ignorant.
The numbers themselves that are obtained during this transformation are called hailstones, because, like hail in the clouds, the numbers then fall, then rise, but sooner or later, all fall to one, at least so it is believed. For convenience, we can make an analogy that the values entered into this algorithm are altitude above sea level. So, if you take the number 26, then it first sharply decreases, then rises to 40, after which it drops to 1 in 10 steps. Here you can give a series for 26:
26 – 13 – 40 – 20 – 10 – 5 – 16 – 8 – 4 – 2 – 1
However, if we take the neighboring number 27, it will jump at a variety of heights, reaching the mark of 9,232, which, continuing the analogy, is higher than Mount Everest, but even this number is destined to collapse to the Ground, although it will take 111 steps to reach 1 and get stuck in the same loop. The same interesting numbers can be numbers 31, 41, 47, 54, 55, 62, 63, 71, 73, 82 and others. For comparison, we can analyze the table (Table 1) and the graph (Fig. 1) for these interesting numbers.
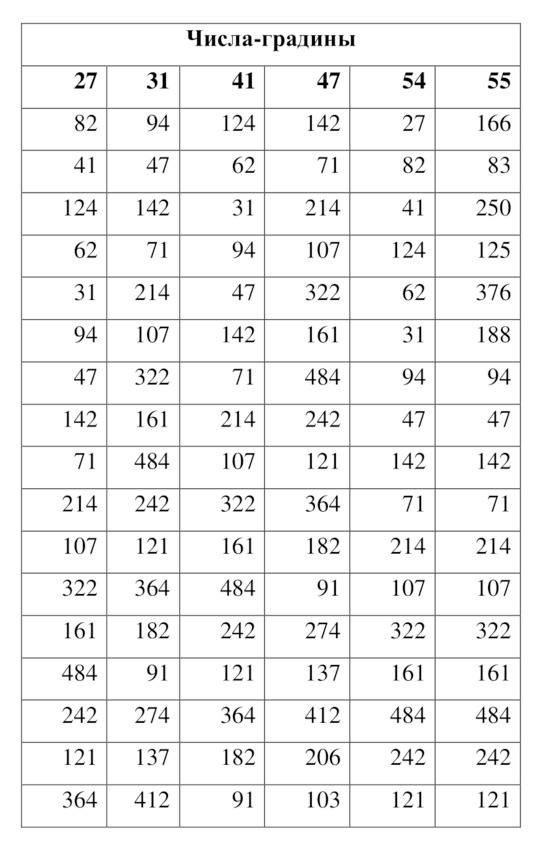
Table 1. A series of long numbers for interesting values of numbers-granules (the first line is the original value)
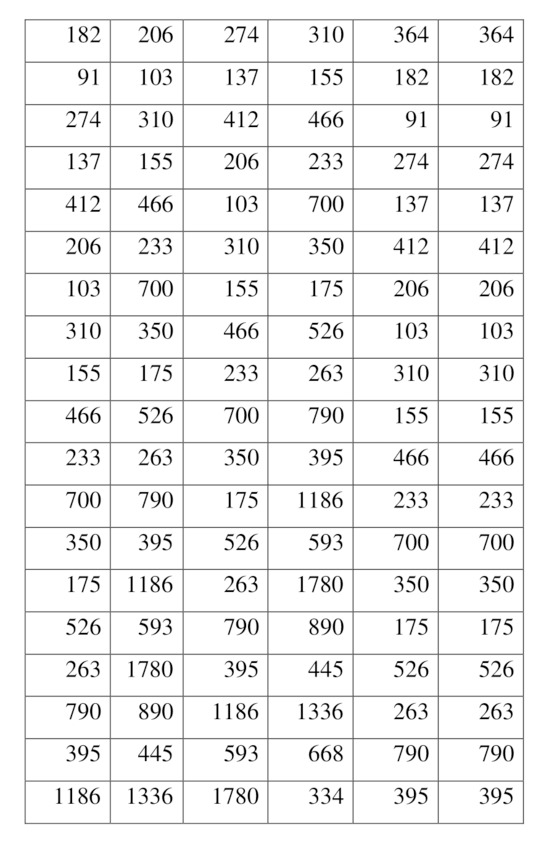
Table 1. A series of long numbers for interesting values of numbers-granules (the first line is the original value)
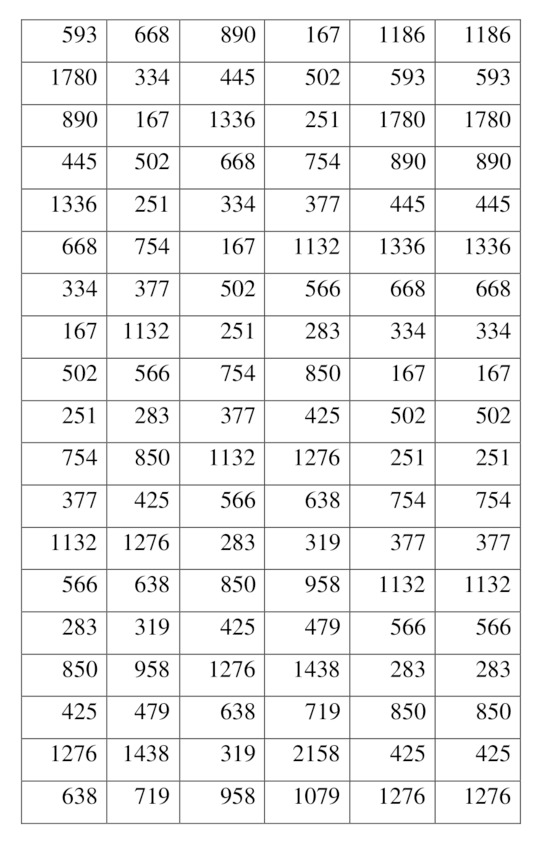
Table 1. A series of long numbers for interesting values of numbers-granules (the first line is the original value)
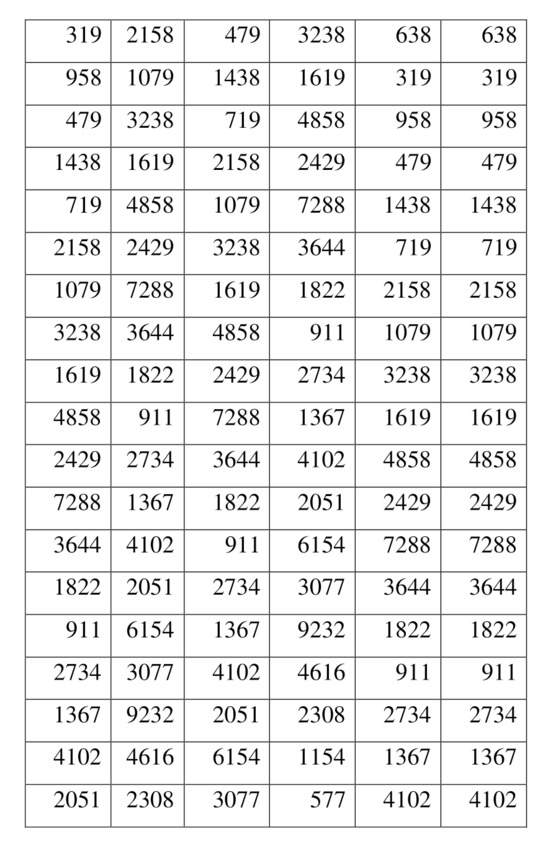
Table 1. A series of long numbers for interesting values of numbers-granules (the first line is the original value)
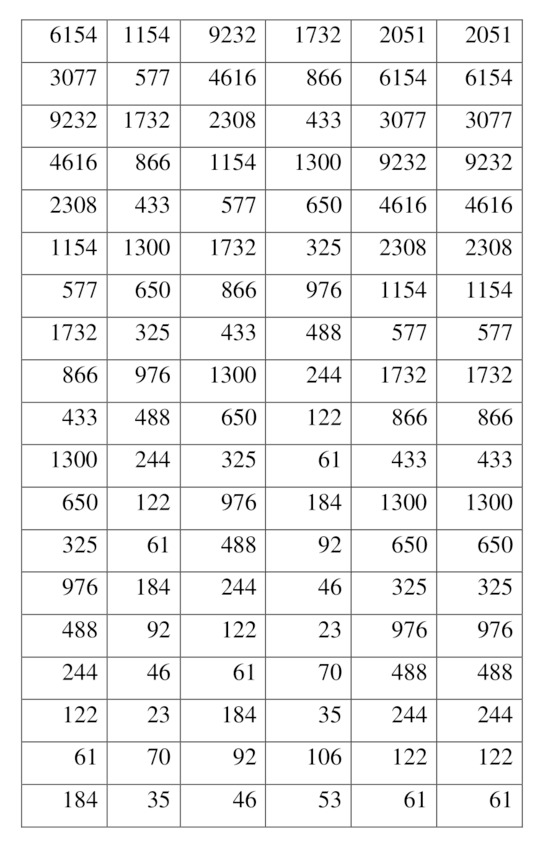
Table 1. A series of long numbers for interesting values of numbers-granules (the first line is the original value)
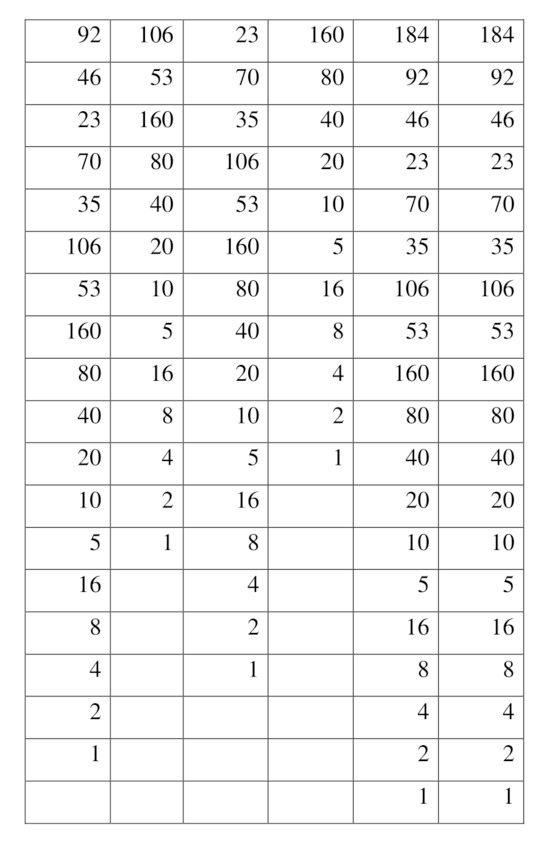
Table 1. A series of long numbers for interesting values of numbers-granules (the first line is the original value)
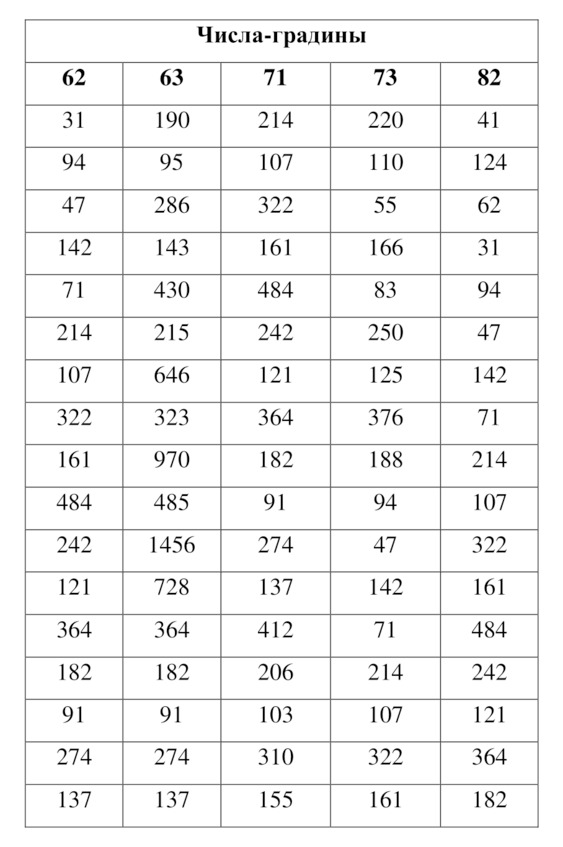
Table 1. A series of long numbers for interesting values of numbers-granules (the first line is the original value)
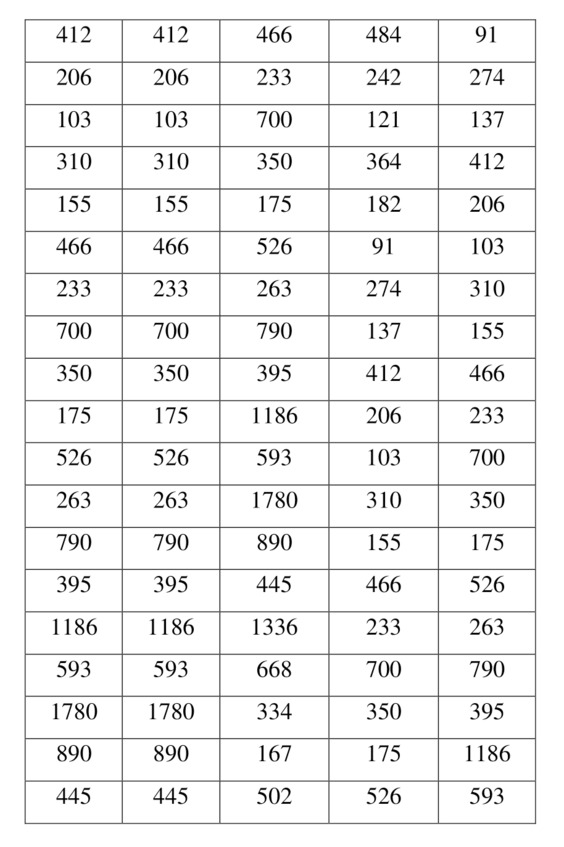
Table 1. A series of long numbers for interesting values of numbers-granules (the first line is the original value)
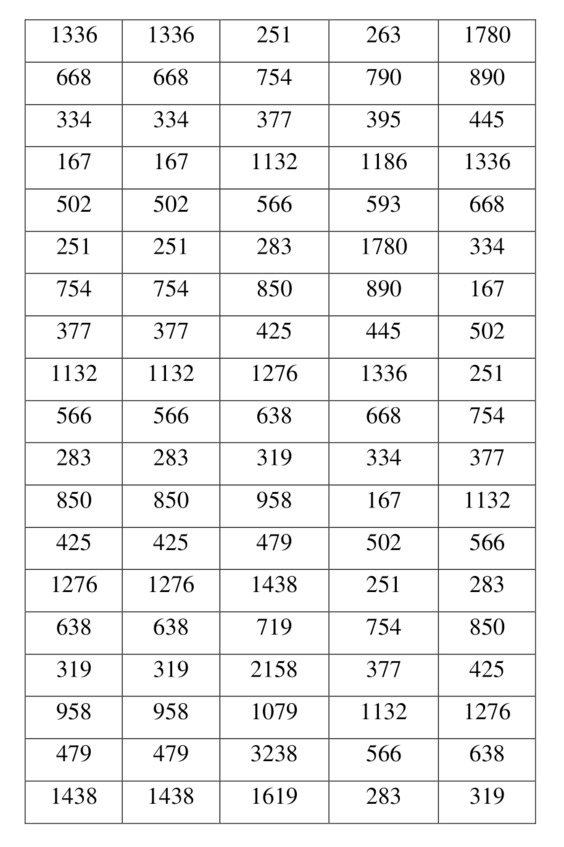
Table 1. A series of long numbers for interesting values of numbers-granules (the first line is the original value)
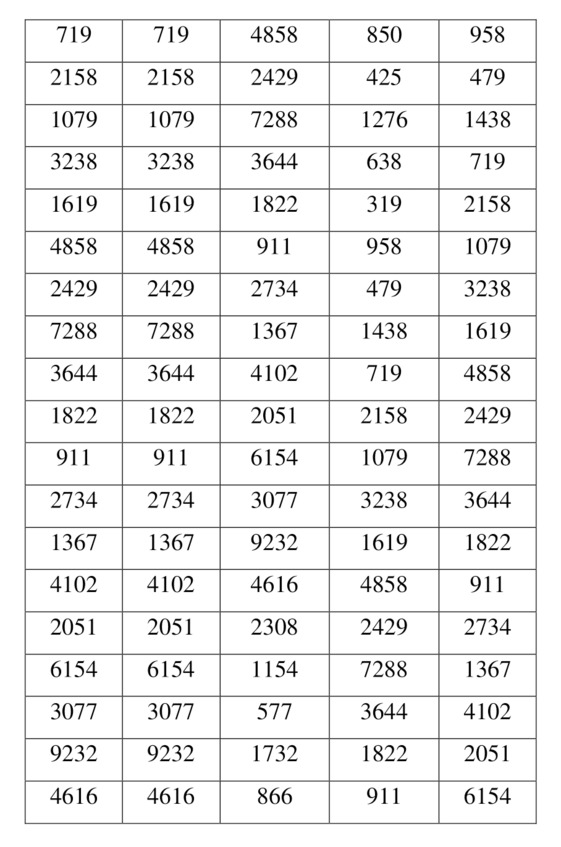
Table 1. A series of long numbers for interesting values of numbers-granules (the first line is the original value)
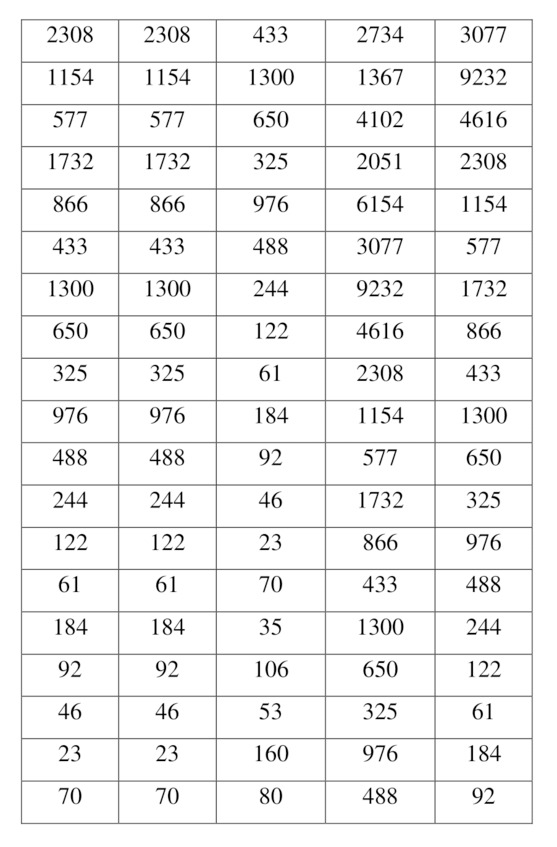
Table 1. A series of long numbers for interesting values of numbers-granules (the first line is the original value)

Table 1. A series of long numbers for interesting values of numbers-granules (the first line is the original value)



